|
|
|
|
Polynomial and/or Polynomial
Functions and Equations |
Graphing
polynomial functions |
|
Zero polynomial |
|
Constant function |
|
Linear function |
|
Quadratic function
and equation |
|
Transition of the
quadratic polynomial from the general to source form and vice versa |
|
The
zeros or the roots of the quadratic function |
|
Vertex (the turning
point, maximum or minimum) - coordinates of translations |
|
Graphing the
quadratic function example |
|
|
|
|
|
|
| Graphing
polynomial functions |
| Polynomial
functions are named in accordance to their degree. |
| Zero polynomial
f
(x)
= 0 |
| The
constant polynomial f
(x)
= 0
is called the zero polynomial and
is graphically represented by the x-axis. |
| Constant function
f (x)
= a0 |
| A
polynomial of degree 0, f
(x)
= a0,
is called a constant function,
its
graph is a horizontal line with |
| y-intercept
a0. |
 |
|
| Linear
function y
= a1x
+ a0 |
| y
= a1x
+ a0
or y
= a1(x
-
x0)
or y
-
y0
= a1x, |
| where
x0
=
-
a0/a1
and/or y0
= a0. |
| By
setting x0
= 0 or y0
= 0
obtained is |
| the
source linear
y
= a1x. |
|
|
 |
|
|
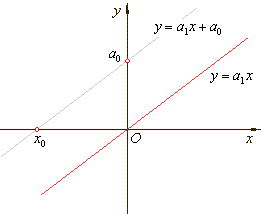| Quadratic
function
y
=
a2x2
+
a1x + a0 |
| 1)
Let calculate the
coordinates of translations of quadratic function using the
formulas, |
| substitute
n
= 2 in |
 |
|
|
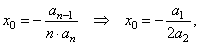| then |
 |
|
|
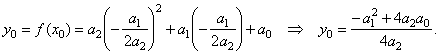| 2)
To
get the source quadratic function we should plug the coordinates
of translations (with changed signs) |
|
into the general form
of the quadratic,
i.e., |
 |
| after
expanding and reducing obtained is |
|
y
=
a2x2
the source quadratic function. |
| 3)
Inversely, by plugging the coordinates of translations into the source quadratic function |
|
y
-
y0
= a2(x
-
x0)2, |
|
|
| and
after
expanding and reducing we obtain |
|
y
=
a2x2
+ a1x
+ a0
the quadratic function
in the general form. |
| ƒ(x)
=
y
=
a2x2
+
a1x + a0
or y
-
y0
= a2(x
-
x0)2, |
| where |
 |
|
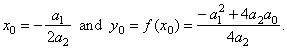| By
setting x0
= 0 and y0
= 0
obtained is |
| the
source quadratic
y
= a2x2
. |
|
| If
a2
·
y0
<
0
then
the roots are |
 |
|
|
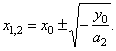The turning point V(x0
, y0
).
|
|
 |
|
|
| Example:
Find zeros and vertex of
the quadratic function y
=
-
x2
+ 2x
+
3
and sketch its graph. |
| Solution:
A quadratic function can
be rewritten into translatable form y
-
y0
= a2(x
-
x0)2
by completing the square, |
|
y
=
-
x2
+ 2x
+ 3 |
Since a2
· y0
<
0 given
quadratic function must have two different real zeros. |
|
y
=
-
(x2
-
2x)
+ 3 |
To find zeros of a function, we set
y
equal to zero and solve for x.
Thus, |
|
y
=
-
[(x
-
1)2
-
1]
+ 3 |
-
4
=
-
(x
-
1)2 |
| y -
4
=
-
(x
-
1)2 |
(x
-
1)2
=
4 |
| y
-
y0
= a2(x
-
x0)2 |
x
-
1
=
±
Ö4 |
| V(x0,
y0)
=> V(1,
4) |
x1,2
=
1
±
2, => x1
= -
1
and x2
= 3. |
|
 |
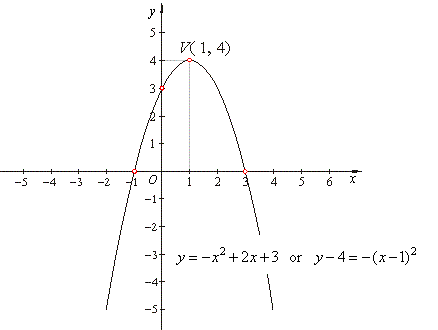| We
can deal with given quadratic using the property of the
polynomial shown above. Thus, |
| 1)
calculate the coordinates of translations of the quadratic
y
=
f (x) =
-
x2
+ 2x
+
3 |
 |
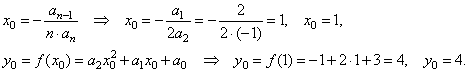| 2)
To
get the source quadratic function, plug the coordinates
of translations (with changed signs) |
|
into the general form
of the quadratic, i.e., |
| y
+ y0
= a2(x
+ x0)2
+ a1(x
+ x0)
+ a0
=>
y
+ 4
= -
(x
+ 1)2
+ 2(x
+ 1)
+
3 |
|
y
=
-
x2
the source quadratic function |
| 3)
Inversely, by plugging the coordinates of translations into the source quadratic function |
| y
- y0
= a2(x
- x0)2
=>
y
- 4
= -
(x -
1)2
|
|
obtained is given quadratic in general form
y
=
-
x2
+ 2x
+
3. |
|
|
|
|
|
|
|
|
|
|
|
| Pre-calculus contents
E |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
