|
| A
revealing insight into the polynomial function -
continue |
|
|
|
|
|
|
|
| The principle provides full control over the polynomial |
| Hence, by
substituting coordinates of translations into the source
polynomial function we can move its graph to any desired
position on the coordinate plane. |
| Since an nth
degree polynomial with
real coefficients can be expressed as a product of its leading
coefficient an
and
n
linear factors of the form (x
- ri),
where ri
denotes its real root and/or complex root |
| f
(x)
= anxn
+ an -
1xn
-
1
+
. . . +
a1x
+ a0
= an(x
- r1)(x
- r2)
. . . (x
- rn), |
| the above expression shows
following coefficients and roots relations called Vieta's
formulas. |
| Therefore, if the leading coefficient an
= 1
we can write for example, |
| the quadratic
function shown by its roots |
| f
(x)
= x2
+ a1x
+ a0
= (x
- r1)(x
- r2)
=
x2
- (r1
+ r2)x
+ r1r2, |
| the
cubic function expressed by roots |
| f
(x)
= x3
+
a2x2
+ a1x
+ a0
= (x
- r1)(x
- r2)(x
- r3)
or |
| f
(x) =
x3
- (r1
+ r2
+ r3)x2
+ (r1r2
+ r1r3
+ r2r3)x
- r1r2r3, |
| the quartic
function wrtten by roots |
| f
(x)
= x4
+
a3x3
+
a2x2
+ a1x
+ a0
= (x
- r1)(x
- r2)(x
- r3)(x
- r4)
or |
|
f (x) =
x4
- (r1
+ r2
+ r3
+ r4)x3
+ (r1r2
+ r1r3
+ r1r4
+ r2r3
+ r2r4
+ r3r4)x2
- |
|
- (r1r2r3
+ r1r2r4
+ r1r3r4
+ r2r3r4)x
+ r1r2r3r4, |
| and so on. |
| Note that the coefficients of a polynomial are expressed as the
alternating sums
of corresponding combinations of
products of roots. |
|
| Example: Let
write the cubic
polynomial whose roots are r1
= 1 and r2,3
= 1 ±
i assuming its
leading coefficient a3
= 1,
and find its source function
and draw their graphs. |
| Then, find the cubic
function obtained by moving the source function by xt
= -
1 and yt
= 2, and draw the graph of
the translated cubic function. |
| Solution:
We write the cubic polynomial
given by its roots to calculate its coefficients, since |
|
f
(x)
= x3
- (r1
+ r2
+ r3)x2
+ (r1r2
+ r1r3
+ r2r3)x
- r1r2r3, |
| then
a2
=
- (r1
+ r2
+ r3)
=
- [1
+ (1 + i)
+ (1 - i)]
=
- 3, |
|
a1
=
r1r2
+ r1r3
+ r2r3
=
1 ·
(1 + i)
+ 1
·
(1 - i)
+ (1 + i)
·
(1 - i)
=
4, |
|
a0
=
-
r1r2r3
=
-
1 ·
(1 + i)
·
(1 - i)
=
- 2, |
| therefore
f (x)
= x3
- 3x2
+ 4x
- 2
or f
(x)
= (x
-
1)[x
- (1 + i)][x
- (1
- i)]. |
| To
find the source form, fs(x)
=
a3x3
+ a1x
of the general cubic polynomial
we calculate coordinates
of translations x0
and y0, |
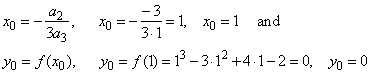 |
| and plug into
y
+ y0
= a3(x
+ x0)3
+
a2(x
+ x0)2
+
a1(x
+ x0)
+
a0 |
|
thus,
y
= (x
+
1)3
- 3(x
+ 1)2
+ 4(x
+ 1)
- 2
= x3
+ x |
|
or fs(x)
= x3
+ x
- the source cubic function of the type 2/1 since, a3a1
> 0. |
| The obtained source
cubic we then move to new position by
plugging the given coordinates of translations, |
| xt
= -1 and yt
= 2,
into y
-
yt
=
a3(x
-
xt )3
+
a1(x
-
xt
) |
| thus,
y
-
2
=
(x
+
1)3
+
(x
+
1)
or y
=
x3
+
3x2
+ 4x
+ 4 |
|
as shows the below figure. |
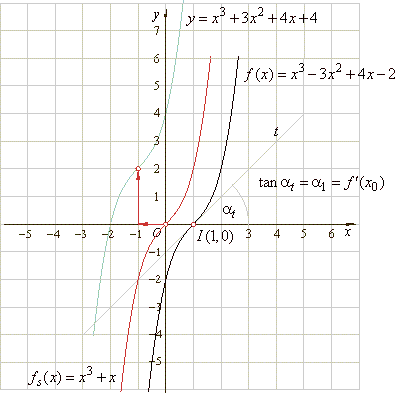 |
|
|
|
|
|
|
| *
For additional information the author invites an interested
visitor to visit the Register
page. |
|
|
|
|
|
|
|
|
|
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |