|
|
|
|
Series |
 Infinite series
Infinite series |
|
The sequence of partial
sums |
|
The
sum of the series |
|
Convergence
of infinite series |
|
Divergence
of infinite series |
|
Convergent
and divergent series
examples |
|
|
|
|
|
|
|
| Infinite series |
| An
infinite series is the sum of infinite sequence of terms which we
denote |
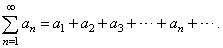 |
| That
is, given an infinite sequence of real numbers, a1,
a2, a3, . . . , an,
. . .
all of the terms of which are added
together, where an
denotes the general term of the series. |
|
| The
sequence of partial
sums |
| Recall
that we've already dealt with infinite series in previous section when
representing real numbers as infinite
decimals. Thus, for example the real number |
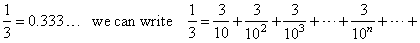 |
| so,
when adding up this infinite number of terms, we start by calculating
its partial sums |
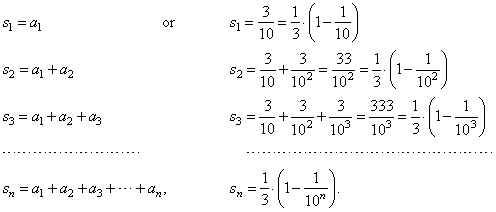 |
| Thus,
obtained is sequence {sn}
of the partial sums, s1,
s2, s3, . . . , sn,
. . .
where sn
denotes the sum of
the first n
terms of the series and is called the nth
partial sum. |
| Therefore,
the
limit of the sequence {sn}
as n
tends to infinity, i.e., |
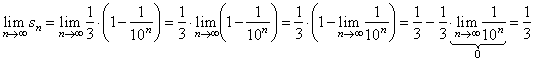 |
|
equals the value of the real number 0.333
. . .
that is, equals the sum of
the infinite series. |
|
|
The
sum of the series |
| An infinite series has a sum if the sequence of its partial sums
converge to a finite number s,
i.e., |
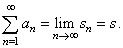 |
| The sum of
an infinite series is the limit of its partial sums
as n
tends to infinity. |
| Let
rewrite the above example using this notation, |
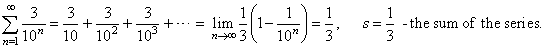 |
| where, |
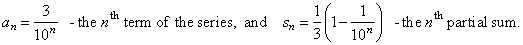 |
|
|
| Convergence
of infinite series |
| If
the
limit of the sequence of partial sums
exists
as a real number, then the series is convergent. |
|
| Divergence
of infinite series |
| If
the limit of the sequence of partial sums does not exist or tends
to +
oo
or -
oo, |
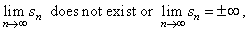 |
| then, the series is
divergent or oscillates. |
|
| Convergent
and divergent series
examples |
| Example: Show
that the series |
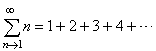 |
diverges. |
|
| Solution:
The given
infinite sum of natural numbers is called the arithmetic series. |
| Using
the formula for the sum
of the arithmetic sequence, whose difference d
= 1,
we calculate the sum of the first n
terms of the series. |
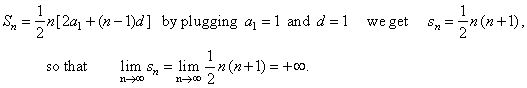 |
|
| Example: Let
show
that the series |
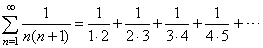 |
converges. |
|
| Solution:
We
use the method of partial
fraction decomposition to rearrange given expression. |
| Since
the denominator consists of linear factors then, we can write |
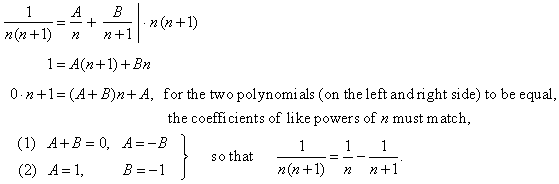 |
| Therefore, |
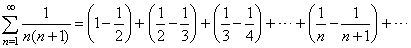 |
|
| Since
the second and the first term of any two successive parentheses cancel
then,
the sum of
the first n
terms of the series |
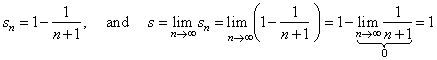 |
|
therefore the series converge, and its sum s
= 1. |
|
|
|
|
|
|
|