|
|
Power
series |
Maclaurin and Taylor series |
|
The power series expansion of the sine function |
|
Properties
of the power series expansion of the sine function |
|
The power series expansion of the cosine function |
|
Properties
of the power series expansion of the cosine function |
|
|
|
|
|
|
| Maclaurin and Taylor series |
| Consider
the polynomial function |
|
f (x) = an
xn
+ an -
1xn -
1 + · · · + a3 x3
+ a2 x2 + a1 x
+ a0.
|
| If
we write the value of the function and the values of its successive
derivatives, at the origin, then |
| f
(0) =
a0, f '(0) = 1· a1,
f ''(0) = 1· 2a2,
f '''(0) = 1· 2· 3a3, . . . , f (n)
(0) =
n! an |
| so we
get the coefficients; |
 |
|
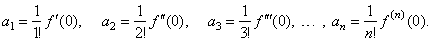| Then,
the polynomial
f (x)
with infinitely many terms, written as the power series |
 |
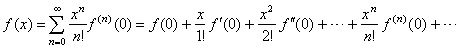and |
|
 |
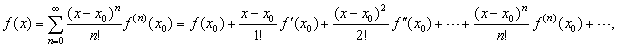| where
0! = 1,
f (0)(x0)
= f(x0)
and
f (n)(x0)
is the nth
derivative of f at
x0, |
|
represents an infinitely differentiable function
and is called Maclaurin series and Taylor series respectively. |
|
| The power series expansion of the
sine function |
| Example: Let
represent the sine function f(x)
= sin x
by the infinite polynomial (or power series). |
| Solution: The
sine function is the infinitely differentiable function defined
for all real numbers. |
| We
use the polynomial with infinitely many terms
|
 |
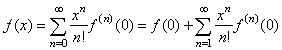| to
represent the sine function. We should calculate the function
value f (0),
and some successive derivatives of
the sine function, to determine the nth
order derivative, therefore
|
 |
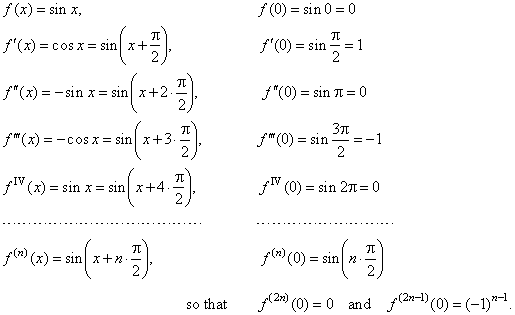| Obtained
values f (0)
and f (n)
(0)
substituted into Maclaurin's formula yield, |
 |
|
|
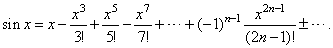Properties
of the power series expansion of the sine function |
| The polynomials that describe the sine function
all are
source polynomials of odd degree. Meaning, both |
| coordinates
of translations |
 |
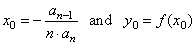are
zero since all even derivatives are |
|
| zero, the infinite polynomial is missing
every next even term, therefore an-1
= 0. |
 |
 |
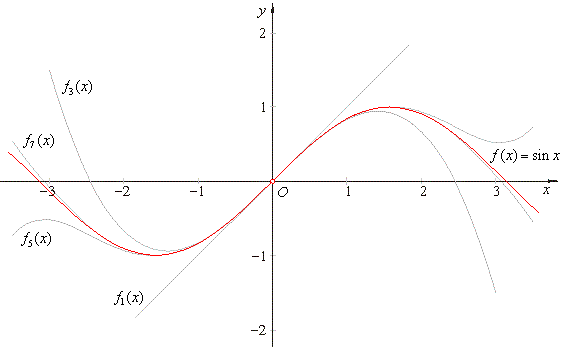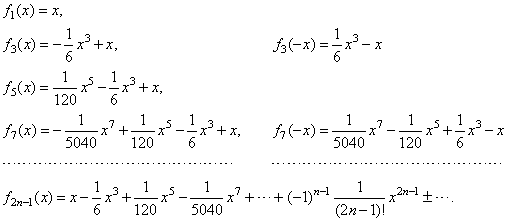| Notice
that every second polynomial in the above sequence, whose leading term
is negative, represents the variant f
(-x)
(or - f
(x))
of the source polynomial of odd degree whose x
or y
variable changed the sign, as are
the graphs, f3
(x)
and f7 (x). |
|
| The power series expansion of the
cosine function |
| Example: Let
represent the cosine function f
(x)
= cos x
by the infinite polynomial (or power series). |
| Solution: The
cosine function is the infinitely differentiable function defined
for all real numbers. |
| We should calculate the function
value f (0),
and some successive derivatives of
the cosine function, to determine the
nth
order derivative, therefore
|
 |
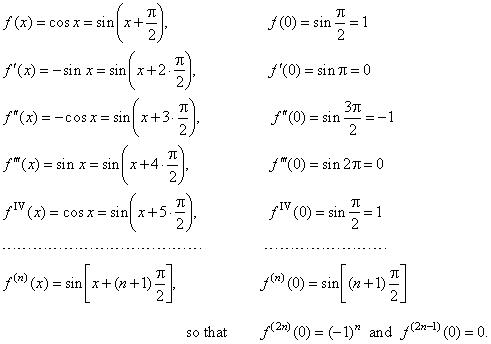| Obtained
values f (0)
and f (n)
(0)
substituted into Maclaurin's formula yield, |
 |
|
|
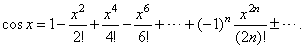Properties
of the power series expansion of the cosine function |
| Since all odd derivatives are zero, the infinite polynomial is missing
every next odd term, therefore an-1
= 0 |
| and the coordinates
of translations |
 |
|
|
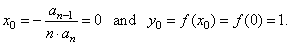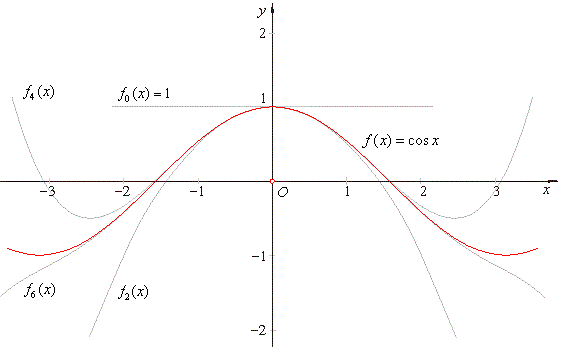
| The polynomials that describe the
cosine function
all are
source polynomials of even degree translated in the direction
of the y
axis by y0
= 1, as is shown in the picture below.
|
 |
 |
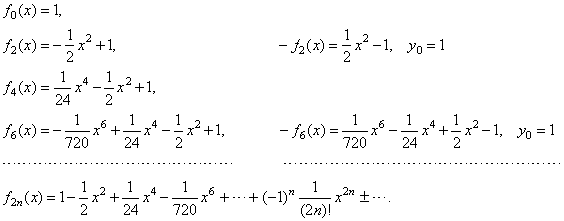| Notice
that every second polynomial in the above sequence, whose leading term
is negative, represents the variant - f
(x)
of the source polynomial of even degree, as are
the graphs, f2
(x)
and f6 (x). |
|
|
|
|
|
|
|
|
|
|
|
| Calculus
contents B |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
