|
| Integral
calculus |
|
|
|
The
indefinite integral |
 Integrating
rational functions
Integrating
rational functions
|
|
The
Ostrogradsky method of the integration of a proper rational
functions
|
|
The
Ostrogradsky method of the integration of a proper rational
functions, examples
|
|
|
|
|
|
|
|
|
|
|
|
Integrating
rational functions
|
|
Recall
that a rational function is a ratio of two polynomials
written Pn(x)
/ Qm(x).
|
|
To
integrate a rational function
|
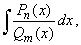
|
|
in
case n >
m, that is, if the
degree of P
is greater than or equal to degree of Q,
we first divide Pn(x)
by Qm(x)
to obtain the sum of the polynomial Pn
-
m(x)
and a proper or simple rational function.
|
|
Then,
we integrate proper rational function using decomposition of rational function
into a sum of partial fractions.
|
|
|
|
The
Ostrogradsky method of the integration of a proper rational
functions
|
|
The
integration of a rational fraction whose denominator Q(x)
has multiple or repeated roots.
|
|
If
P(x)
and Q(x)
are polynomials with real coefficients and P(x)
/ Q(x)
is a proper fraction, and Q(x)
has multiple
roots, then
|
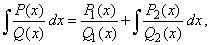
|
|
where
Q1(x)
is the greatest common divisor of Q(x)
and its derivative Q'
(x),
while
|
|
Q2(x)
= Q (x) / Q1(x).
|
|
Undetermined
coefficients of he polynomials P1(x)
and P2(x),
whose
degrees are one less than of the polynomials
Q1(x)
and Q2(x)
respectively, we calculate by deriving the above integral
identity.
|
|
|
|
The
Ostrogradsky method of the integration of a proper rational
functions, examples
|
|
|
|
Since
Q(x)
= (x3 + 1)2
and Q'
(x) = 6x2(x3 + 1)
then Q1(x)
= x3 + 1
is the greatest common divisor of Q(x)
and Q'
(x),
and Q2(x)
= Q (x) / Q1(x)
= x3 + 1.
Therefore,
|
|
|
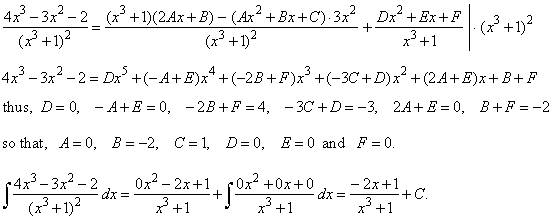
|
|
|
|
|
|
Since
Q(x)
= (x + 1)2 · (x2 + 1)2
and Q'
(x) = 2(x + 1)
· (x2 + 1)2
+
4x(x + 1)2
· (x2 + 1)
or
|
|
Q'
(x) = 2(x + 1)(x2 + 1)[(x2 + 1)
+ 2x(x + 1)]
then Q1(x)
= (x + 1) (x2 + 1)
and
|
|
Q2(x)
= Q (x) / Q1(x)
= (x + 1) (x2 + 1).
Therefore,
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Calculus contents
F |
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |