|
| Integral
calculus |
|
|
|
The
indefinite integral |
 Integrating
irrational functions
Integrating
irrational functions
|
|
Integration of
irrational functions, examples
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Integrating
irrational functions
|
|
|
|
where,
R
is a rational function and, p1,
q1,
p2,
q2,
. . . are integers,
|
| we
can solve using substitution |
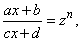 |
|
|
where
the power n
is the least common multiple of q1,
q2,
. . . .
|
|
|
|
Integration of
irrational functions examples
|
|
|
|
|
|
|
|
|
|
|
|
Note
that here we used the Ostrogradsky method and the solution of I2
already evaluated, see above.
|
|
|
| Integration of
irrational functions of the form |
|
|
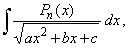 |
|
|
where
Pn(x)
is an n-th
degree polynomial.
|
|
|
|
where
Qn -
1(x) is an (n
-
1) -th
degree polynomial of undetermined
coefficients and l
is a constant.
|
|
Coefficients
of the polynomial Q
and the constant l
we obtain by deriving the above identity.
|
|
|
|
|
|
|
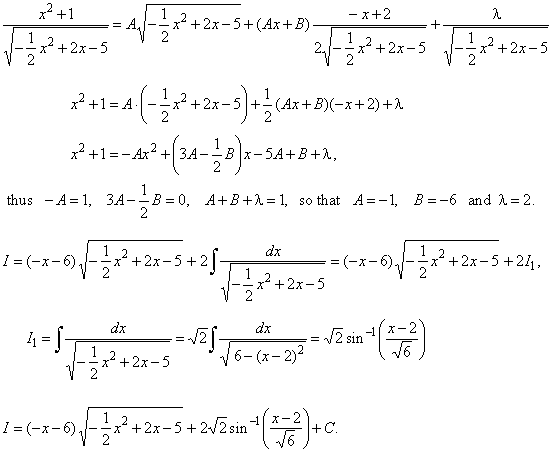
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Calculus contents
F |
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |