|
| Integral
calculus |
|
|
|
|
The
definite integral |
|
Riemann
sum |
 Calculating
a definite integral from the definition
Calculating
a definite integral from the definition |
|
Calculating
a definite integral from the definition, examples |
|
|
|
|
|
|
|
| Riemann
sum |
| Until
now, in the definition of the sums, S
and s
we've used the maximum and the minimum values, Mi
and mi
of a given continuous function
f, so that mi
< f (x)
< Mi for x
in [xi
-
1, xi],
i = 1, 2 , . . . , n. |
| Now,
if we arbitrarily choose a point xi'
in every interval Dxi
and make products f (xi' )
Dxi,
then |
| mi
Dxi
<
f (xi' )
Dx
< Mi
Dxi,
i = 1, 2 , . . . , n |
| and
by adding up |
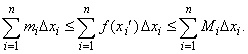 |
| The
left-hand and right-hand sides of the above inequality are the sums, s
and S
respectively that, because of continuity of f,
tend to the same limit value I
when the number
of subintervals n
increases to infinity, such that the length of
every interval Dxi
tends to zero, for any partition of the interval [a,
b]
and arbitrarily chosen
points xi'
in the subintervals [xi
-
1, xi].
Hence,
the middle term |
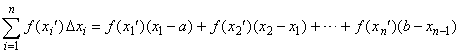 |
| called
a Rieman sum, will tend to the same limit value.
|
| Therefore,
if f
is a positive continuous function on the interval [a,
b] then, the definite
integral of the function from a
to b
is defined to be the limit
|
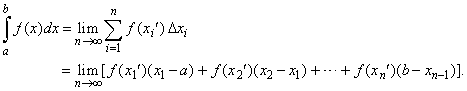 |
| Note
that the limit value of the sum changes as the number
of subintervals n
increases to infinity while the length
(Dxi)
of each tends to zero, for any partition of the interval [a,
b].
|
|
| Calculating
a definite integral from the definition |
|
As the sequence of inscribed rectangles s
tends to the definite
integral increasingly while the sequence of circumscribed rectangles S
tends to the same value decreasingly then |
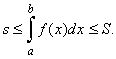 |
| Thus,
we
can approximate the area under the graph of a function over the interval
[a,
b]
to any desired level of accuracy using
the Riemann sum of inscribed or circumscribed rectangles. |
| The
area of the ith
rectangle f (xi' )
Dxi,
denoted as height times base, represents the ith
term of Riemann sum
and is called the element of area. |
| When
we use the partition of the interval [a,
b] into n
equal subintervals (regular partition) then |
 |
| be
the length of the intervals [xi
-
1, xi],
i = 1, 2 , . . . , n. |
|
| Calculating
a definite integral from the definition, examples |
| Example:
Evaluate |
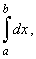 |
where f (x)
=
1, using the
definition of the definite integral. |
|
| Solution: Since
the graph of the constant f (x)
=
1
is the line passing through the point (0,
1)
parallel to the x-axis,
the region under the graph is the rectangle of
the base
=
(b
-
a)
with the height h
=
1. |
| Thus,
the area |
| A
=
(b
-
a) · 1
=
b
-
a, |
| as
shows the right figure. Therefore, |
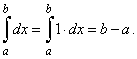 |
|
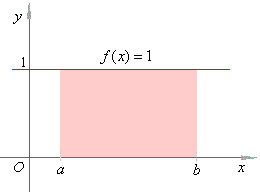 |
|
|
| Example:
Evaluate |
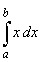 |
where f (x)
=
x, using the
definition of the definite integral. |
|
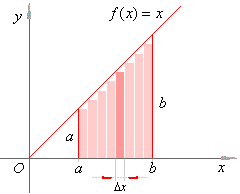
| Solution: Since
the graph of f (x)
=
x
is the line through the origin, coordinates of every its point y
=
x,
so
the region under the graph is the trapezium
with the height
b
-
a
and whose parallel sides are
a
and b. |
| Let's
use the partition of the interval [a,
b] into n
equal subintervals,
so that Dx
=
(b
-
a) / n
and calculate |
| the
lower sum s
of inscribed rectangles, as
shows the right |
| figure.
If
we choose the point xi'
to be the left-hand end of |
| each
subinterval,
then |
| x1'
=
a
and
f (x1'
)
=
a |
| x2'
=
a
+
Dx,
f (x2'
)
=
a
+
Dx |
|
x3'
=
a
+
2Dx,
f (x3'
)
=
a
+
2Dx |
|
·
· ·
·
· · |
| xn'
=
a
+
(n
-
1)Dx,
f (xn'
)
=
a
+
(n
-
1)Dx. |
|
 |
|
| We
use the Riemann sum to calculate the sum of inscribed rectangles with
bases of the same length, |
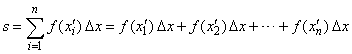 |
|
therefore, s
=
a
Dx
+
(a
+
Dx)Dx
+
(a
+
2Dx)Dx
+
·
· ·
+
[a
+
(n
-
1)Dx]Dx |
|
s
=
Dx[n
a +
Dx(1
+
2
+
·
· ·
+
(n
-
1))] |
| to
calculate the sum of natural numbers inside of square brackets we use
the formula |
| Sn
= [2a1 + (n -
1)d] for the sum of the
arithmetic sequence whose first term a1
= 1 and difference d
= 1, |
| so
we get the sum equals
(n
-
1)n
/ 2, and since Dx
=
(b
-
a) / n
then, |
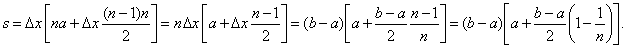 |
| Thus
the area under the graph of f (x)
=
x
over the interval [a,
b] |
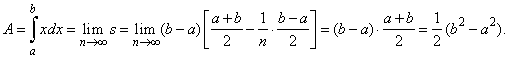 |