|
|
|
ALGEBRA
- solved problems
|
|
|
|
|
|
|
|
|
 Factoring algebraic
expressions
Factoring algebraic
expressions |
| Factoring
algebraic expression by finding (determining) a common factor |
|
| 84. |
Factorize given expressions.
|
|
|
Solutions:
|
|
a) 3x
- 6y
= 3 · (x
- 2y),
b) xy
- y2
= y ·
(x - y),
c) a
-
a2
= a · (1 -
a), |
|
|
d) x3
-3x2
+
x
= x · (x2
- 3x
+1),
e) x(a
+
b)
- (a
+
b)
= (a
+
b)
· (x
-
1), |
|
|
f)
a(x
- 3y)
- x
+
3y =
a(x -
3y) - (x
- 3y)
= (x - 3y)
· (a
- 1). |
|
|
|
|
Grouping
like terms, grouping and factorizing four terms
|
|
|
| 85. |
Factorize given expressions.
|
|
|
Solutions:
|
|
a) ax
- bx
- a
+
b
= x(a
-
b)
- (a
-
b)
= (a
-
b)
·
(x
-
1), |
|
|
b) a
- 1
- ab
+
b
= (a
- 1)
- b
·
(a
- 1)
= (a
- 1)
· (1 -
b), |
|
|
c) x2
+
ax
- bx
- ab
= x(x
+
a)
- b
·
(x
+
a)
= (x +
a)
· (x
-
b), |
|
|
d)
5ab2
- 3a3
- 10b3
+ 6a2b
= 5b2(a -
2b)
-3a2(a
- 2b)
= (a - 2b)(5b2
- 3a2). |
|
|
|
|
The
square of a binomial - perfect squares trinomials
|
|
| 86. |
Factorize given expressions.
|
|
|
Solutions:
|
|
a) 1
- 4x
+ 4x2
= 12 -
2 ·
2x
+ (2x)2
= (1 - 2x)2
= (1 - 2x)
· (1 -
2x), |
|
|
b) a5
+ 6a4b
+ 9a3b2
= a3 · (a2
+ 6ab
+ 9b2
) = a3(a
+ 3b)2
= a3(a
+ 3b)(a
+ 3b). |
|
|
| Difference of
two
squares |
|
| 87. |
Factorize given expressions.
|
|
|
Solutions:
|
|
a) 16x2
- 1
= (4x)2
- 12
= (4x
-1)
· (4x
+1), |
|
|
b) 5y3
- 20x2y
= 5y
· (y2
- 4x2)
= 5y
[y2
- (2x)2]
= 5y(y
- 2x)(y
+ 2x), |
|
c) 9x2
-
(x
+ 2)2
= [3x -
(x
+ 2)]
· [3x
+
(x
+ 2)]
= (2x
-2)
·
(4x
+ 2)
= 4(x
-1)
· (2x
+1). |
|
|
| Factoring
quadratic trinomials |
|
| A
quadratic trinomial ax2
+ bx
+ c
can be factorized as |
| ax2
+ bx
+ c
= a·[x2
+ (b/a)·x
+ c/a]
= a·(x
-
x1)(x
-
x2), |
where
x1
+ x2
= b/a and
x1·
x2
= c/a |
|
|
| 88. |
Factorize quadratic trinomials.
|
|
|
Solutions:
|
|
a) x2
- 3x
-10
= x2
+ (-5
+
2)·x
+ (-5)·(+2)
= x2
- 5x
+
2x
-10
= |
|
|
|
= x ·
(x
- 5)
+ 2 ·
(x
- 5)
= (x
- 5)
·
(x
+ 2), |
|
|
|
|
|
b) 2x2
- 7x
+ 3
= 2 ·
(x2
- 7/2x
+ 3/2)
= 2(x2
- 1/2x
- 3x
+ 3/2)
= |
|
|
= 2[x·(x
- 1/2)
- 3·
(x - 1/2)]
= 2·
(x - 1/2)·(x
- 3)
= (2x
- 1)·
(x - 3), |
|
|
|
|
|
c) 3x2
- x
- 2
= 3(x2
- 1/3x
- 2/3)
= 3(x2 +
2/3x
- x
- 2/3)
= |
|
|
= 3[x·(x
+ 2/3)
-
(x + 2/3)]
= 3·(x
+ 2/3)·(x
- 1)
= (3x + 2)·(x
- 1). |
|
|
| 89. |
Given
are leading
coefficient a2
=
-1
and
the complex
roots,
x1 =
1 +
i
and
x2
=
1 - i,
of
a
|
|
| second
degree polynomial, find
the polynomial using
the above theorem. |
| Solution:
|
By
plugging the given values into a2x2
+ a1x
+ a0 = a2(x
- x1)
(x
- x2) |
|
a2x2
+ a1x
+ a0 = -1[x
-
(1
+ i)]
· [x
-
(1 - i)]
= -[(x
- 1)
- i]
· [(x - 1)
+ i] |
|
= -[(x - 1)2
- i2]
=
-(x2
- 2x
+ 1 +
1)
=
- x2
+ 2x
- 2. |
|
|
| Factoring
cubic or a third degree polynomial |
| |
a3x3
+ a2x2
+ a1x
+ a0 = a3(x
- x1)
(x
- x2)
(x
- x3)
= |
|
|
| |
=
a3[x3
-
(x1 +
x2
+
x3)x2
+
(x1x2 +
x1x3
+
x2x3)x
- x1x2x3]. |
|
|
| 90. |
The
real root of the polynomial -
x3
-
x2
+ 4x
- 6
is
x1 =
- 3,
factorize the polynomial.
|
|
| Solution:
|
We
divide given polynomial by one of its known
factors, |
|
a3x3
+ a2x2
+ a1x
+ a0 = a3(x
- x1)
(x
- x2)
(x
- x3) |
|
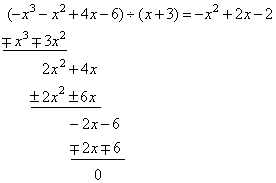 |
|
then
we calculate another two roots of given cubic by solving
obtained quadratic trinomial, |
|
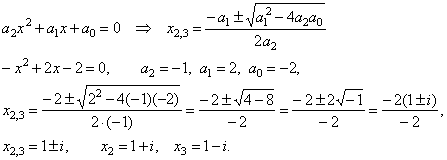 |
|
Finally
we use the theorem to factorize given polynomial (see
the previous example), |
|
a3(x
- x1)(x
- x2)(x
- x3)
= -1(x
+ 3)[x
-
(1
+ i)][x
-
(1 - i)] |
|
= -
(x
+ 3)(x2
- 2x
+ 2). |
|
Notice that given cubic has one real root and the pair of the conjugate complex roots. |
|
Odd
degree polynomials must have at least one real root. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Solved
problems contents |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |