|
|
|
|
Quadratic Equations and Quadratic
Function |
 Quadratic equation
Quadratic equation |
|
Solving quadratic equations by completing the
square, the quadratic formula |
|
Solving quadratic equations by factoring,
Vieta's formula |
| |
|
|
|
|
|
| Quadratic equation |
| A
quadratic equation is a polynomial equation of the second
degree. The general or standard form is |
| ax2
+ bx + c = 0,
a is
not 0 |
| where a,
b
and c
are coefficients. |
|
|
Solving quadratic equations by completing the
square, the quadratic formula |
| The solutions of the equation
are called roots (which may be real or complex) and are given by
the quadratic formula. |
|
We
use the completing the square method to derive the quadratic
formula to find the roots, |
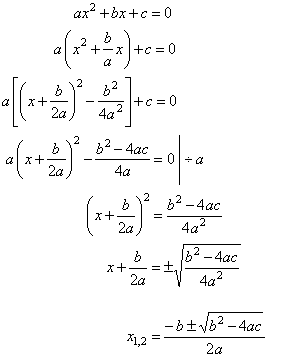 |
| The
roots can also be written |
 |
| If
c = 0,
the roots of the
equation ax2
+ bx = 0
we can obtain by factoring |
|
x(ax
+ b) = 0 |
| or
by using the quadratic formula, so we get x1=
0 and x2
= -b/a. |
|
| Solving quadratic equations by factoring, Vieta’s formula |
| A
quadratic trinomial ax2
+ bx
+ c
can be factorized as |
| ax2
+ bx
+ c
= a·[x2
+ (b/a)·x
+ c/a]
= a·(x
-
x1)(x
-
x2), |
where
x1
+ x2
= b/a and
x1·
x2
= c/a |
|
| That
means, to factor a quadratic trinomial we should find such a
pair of numbers x1
and x2
whose sum equals b/a
and whose product equals c/a. |
| So,
if a >
0 and the constant term c
negative, then the signs of x1
and x2
will be different while, when c
is positive, their signs will be the same. |
|
Then we use zero product principle to solve the quadratic equation,
that is, a product a
· b = 0
if at least one of its factors is 0. |
|
Examples:
Find the roots of each
quadratic equation by factoring:
|
|
a) x2
- 3x
-10
= 0
|
|
since
x2
- 3x
-10
= x2
+ (-5
+
2)·x
+ (-5)·(+2)
= x2
- 5x
+
2x
-10
|
|
= x ·
(x
- 5)
+ 2 ·
(x
- 5)
= (x
- 5)
·
(x
+ 2),
|
| then
x
- 5
= 0
=>
x1 =
5,
or x
+ 2
= 0
=>
x2 =
-2. |
|
| b) 2x2
- 7x
+ 3
= 0 |
|
as 2x2
- 7x
+ 3
= 2[x2
- (7/2)x
+ 3/2]
= 2[x2
- (1/2)x
- 3x
+ 3/2]
= |
|
= 2[x(x
- 1/2)
- 3(x - 1/2)]
= 2
(x - 1/2)(x
- 3)
= (2x
- 1)
(x - 3), |
| then
2x
- 1
= 0
=>
x1 =
1/2,
or x - 3
= 0
=>
x2 =
3 |
|
| c) 3x2
- x
- 2
= 0 |
|
as 3x2
- x
- 2
= 3[x2
- (1/3)x
- 2/3]
= 3[x2 +
(2/3)x
- x
- 2/3]
= |
|
= 3[x(x
+ 2/3)
-
(x + 2/3)]
= 3·(x
+ 2/3)(x
- 1)
= (3x + 2)(x
- 1), |
| then
3x + 2
= 0
=>
x1 =
-2/3,
or x
- 1
= 0
=>
x2 =
1. |
|
|
| Example:
Given
are leading
coefficient a2
=
-1
and
the pair
of conjugate complex
roots, |
|
x1 =
1 +
i
and
x2
=
1 - i,
of
a second
degree polynomial; |
| a)
find
the polynomial using
the above theorem, |
| b)
make a check of the solutions of the polynomial using the
quadratic formula. |
| Solution: |
a)
By
plugging the given values into a2x2
+ a1x
+ a0 = a2(x
- x1)
(x
- x2) |
|
a2x2
+ a1x
+ a0 = -1[x
-
(1
+ i)]
· [x
-
(1 - i)]
= -[(x
- 1)
- i]
· [(x - 1)
+ i]
=
|
|
= -[(x - 1)2
- i2]
=
-(x2
- 2x
+ 1 +
1)
=
- x2
+ 2x
- 2 |
|
b)
Check the polynomial for the roots, |
|
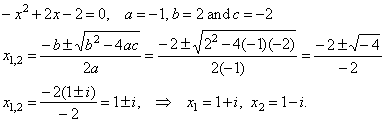 |
|
|
|
|
|
|
|
|
|
|
|
|
|
| Pre-calculus
contents B |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |