|
Example: Fresh figs contain 90%
of water and dry figs contain 12%. How many kilos of dry figs are obtained
|
|
by drying 264 kg of fresh
figs?
|
|
Solution: Using the
proportion B
:
(B
± A)
= 100 :
(100
± p),
|
|
As fresh figs contain 10% of the dry substance and dry figs contain 88% of the dry
substance it follows that
|
| 264 kg of fresh figs contains 26.4 kg of the dry substance.
|
| Therefore, 26.4 kg is 88% of quantity of dry figs or it is
weight of the dry figs decreased by 12% of water, |
| so
B -
A = 26.4 kg,
and by using the above formula |
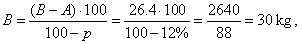 |
| obtained
is the
weight of the dry figs. |
|
|
Example: Sum of all three-digits numbers divisible by 3 is?
|
|
Solution: The three-digits numbers divisible by 3
are;
|
|
102, 105, 108,
. . . , 996, 999
|
| and
they represent the arithmetic
progression |
|
a1,
a2,
a3,
. . . ., an
- 1,
an,
where a1
= 102, an
= 999
and d
= 3, |
| since
an
= a1 + (n - 1)
· d then 999
= 102 + (n - 1)
· 3
|
|
3
· (n - 1)
= 897 | ¸3
|
| n
- 1
= 299
=> n
=
300 |
| Using the
sum formula, |
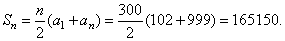 |
|
|
|
Example: How many minutes pass while watch hands coincide again?
|
|
Solution: Suppose watch hands last coincide at noon. They will
coincide again 5 to 6 minutes after 13 hours.
|
| To reach 13 hours, the minute hand traveled full circle 360° or 60 minutes,
and the hour hand traveled 30°.
|
|
Therefore, the minute hand travels at speed of |
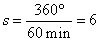 |
degrees per minute, |
|
| while the hour hand travels at speed of |
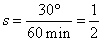 |
degree per minute. |
|
|
Hands will coincide again the minute hand passes additional distance of
30° +
x° while, at the same time, the hour hand passes the distance of
x°, i.e.,
|
|
|
| Thus, watch hands will coincide again after
60 min + 5 min + 5/11 min = 65 and 5/11 min. |
|
|
Example: If
v = g t + v0
and |
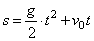 |
then t is
equal?
|
|
|
Solution:
|
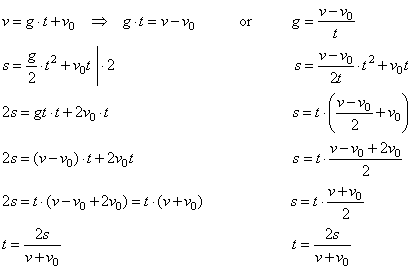
|