|
| Applications
of Trigonometry |
|
|
Oblique
or Scalene Triangle |
|
The tangent law or the tangent rule |
|
Half-angle formulas |
Area of a triangle, the radius of
the circumscribed circle and the radius of the inscribed circle |
|
The radius of the circumscribed circle or circumcircle |
|
The
radius of the inscribed circle |
|
Oblique or scalene triangle
examples |
|
|
|
|
|
|
|
| Oblique
or Scalene Triangle |
|
The tangent law or the tangent rule |
|
Dividing corresponding pairs of Mollweide's formulas and applying following identities, |
 |
|
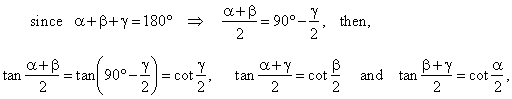obtained are equations that represent the tangent law |
 |
|
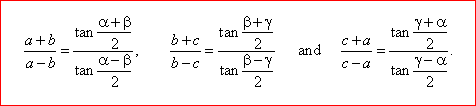| Half-angle formulas |
|
Equating the formula of the cosine law and known identities, that is, |
 |
|
plugged into the above formula gives |
 |
|
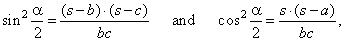dividing above expressions |
 |
|
Applying the same method on the angles, b
and g, obtained are |
 |
|
| Area of a triangle, the radius of the circumscribed circle and the radius of the inscribed circle |
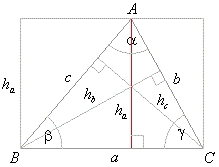
| Rectangular in the
figure below is composed of two pairs of congruent right triangles
formed by the given oblique triangle. Therefore, the area of a triangle equals the half of the rectangular
area, |
|
|
| In the right triangles in the
right diagram, |
| ha
= b · sing,
hb
= c · sina,
hc
= a · sinb, |
| and
by plugging into above formulas for the area |
|
|
|
 |
|
| the
area of a triangle in terms of an angle and the
sides adjacent to it. |
| If, in the above formulas for the area, we substitute each side applying the
sine law, that is |
 |
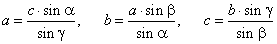| obtained is
the area of a triangle in terms of a side and all its
angles, |
|
|
|
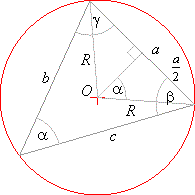
| The radius of the circumscribed circle or circumcircle |
| Using known relation, which states that the angle subtended by a chord at the circumference is half the angle
subtended at the center, from the right triangle in
the below diagram follows, |
|
|
| the radius of the circumscribed
circle,
or |
| a
= 2R · sina,
b
= 2R · sinb,
c
= 2R · sing. |
| Plugging the sides into
A
= (1/2) ab sing
obtained is |
| |
A
= 2R2 · sina
· sinb
·
sing |
|
|
|
 |
|
| the
area of a triangle in terms of the radius of circumcircle and
angles. |
|
| If, in the above formula for the area which includes two adjacent sides and the angle between them, a given
angle is substituted as follows |
 |
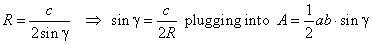| obtained
is |
 |
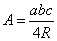the
area of a triangle in terms of sides and the radius of the
circumcircle. |
|
|
| Oblique or scalene triangle
examples |
| Example:
Determine length of sides, angles and area of a triangle of which
a + b
= 17 cm, c
= 15 cm and
angle g =113°. |
| Solution: Using Mollweide's formula |
 |
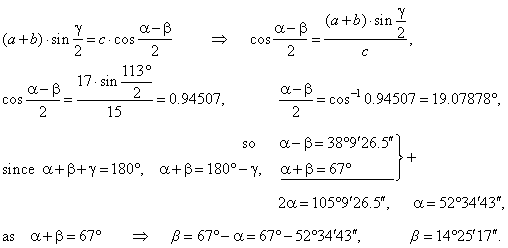| Applying the sine law, |
 |
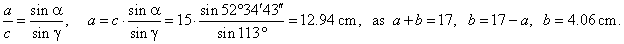| Area of the triangle from the formula |
 |
|
|
|
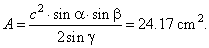| Example:
Given is the sum of the sides of a triangle
a + b +
c = 46 cm, the radius of the incircle
(or
inradius) r =
Ö3
cm and angle
b
=60°. Find all sides and angles of the triangle. |
| Solution: Using
the formula |
 |
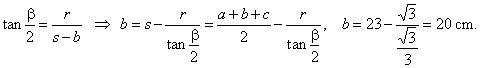| Using Mollweide's formula |
 |
| Applying the sine law, |
 |
|
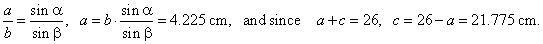| Example:
Determine the area of an isosceles triangle of which, the line segment that joints the midpoint of
one of its equal sides by the midpoint of the base equals the half of the radius
R of the
circumcircle. |
| Solution: In the similar triangles
ABC
and CDE,
b/2 = R/2
=> b = R. |
|
|
| Area of the triangle |
 |
|
|
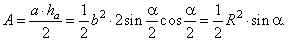Equating obtained formula with the known
formula for the area of a triangle in terms of the radius of the
|
| circumcircle |
 |
|
|
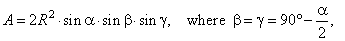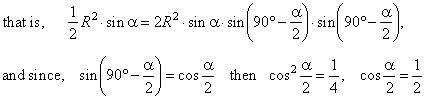
| so, the area of the
triangle |
 |
|
|
|
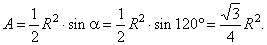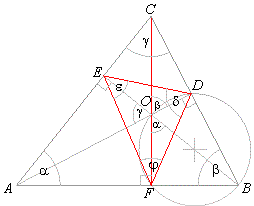
| Example:
Given is a triangle with sides,
a,
b
and
c, and angles,
a,
b
and g. In the triangle inscribed is a
triangle whose vertices lie in foots of the altitudes of the given triangle, as is shown in
the figure down.
|
|
Determine sides, angles and the area of the inscribed triangle.
|
| Solution: Quadrilateral
ODBF is cyclic (since the sum of the opposite
angles is 180°) that is
around which a circle can be circumscribed. |
|
Thus, angle ODF
= angle
OBF
= 90° -
a since they are inscribed angles
subtended by the same arc OF.
|
|
As well, angle ODE
= angle
OCE
= 90° -
a
so angle ODF
+ angle
ODE
= d
= 180° -
2a.
|
| Note that two angles with mutually perpendicular sides are
equal. |
| Also, angle
OFD
= angle
OBD
= 90° -
g |
| since they are inscribed angles
subtended by the same arc OD. |
| As well, angle
OFE
= angle
EAO
= 90° -
g |
|
so angle
OFD
+ angle
OFE
= j
= 180° -
2g. |
| On a similar way can be proved that
e
= 180° -
2b. |
|
 |
|
|
Triangles, BDF
and ABC
are similar since, angle
DFB
= 90° -
angle OFD
= 90° -
(90° -
g)
= g
therefore
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
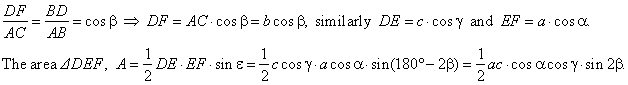
| Geometry
and use of trigonometry contents - B |
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
