Rational Functions
      Basic properties of rational functions
         Vertical asymptotes of rational functions
         Horizontal asymptotes of rational functions
         The oblique or slant asymptote of rational functions
      The graph of the reciprocal function  f (x) = c/x, equilateral or rectangular hyperbola
      Translation of the reciprocal function  f (x) = (ax + b)/(cx + d), linear rational function
         Graphing rational functions examples
Basic properties of rational functions
The functions that most likely have asymptotes are rational functions.
Vertical asymptote
The line x = a is a vertical asymptote of a function  f  if  f (x) approaches infinity (or negative infinity) as x approaches a from the left or right.
So, vertical asymptotes occur when the denominator of the simplified rational function is equal to 0. Note that the simplified rational function has cancelled all factors common to both the numerator and denominator.
Horizontal asymptote
The line y = c is a horizontal asymptote of a function  f  if  f (x) approaches c as x approaches infinity (or negative infinity).
The existence of the horizontal asymptote is related to the degrees of both polynomials in the numerator and the denominator of the given rational function.
Horizontal asymptotes occur when either, the degree of the numerator is less then or equal to the degree of the denominator.
In the case when the degree (n) of the numerator is less then the degree (m) of the denominator, the x-axis
y = 0 is the asymptote.
If the degrees of both polynomials, in the numerator and the denominator, are equal then,  y = an / bm  is the horizontal asymptote, written as the ratio of their highest degree term coefficients respectively.
When the degree of the numerator of a rational function is greater than the degree of the denominator, the function has no horizontal asymptote.
Oblique or slant asymptote
The line  y = mx + c  is a slant or oblique asymptote of a function f  if  f (x) approaches the line as x approaches infinity (or negative infinity).
A rational function will have a slant (oblique) asymptote if the degree (n) of the numerator is exactly one more than the degree (m) of the denominator that is if  n = m + 1.
Dividing the two polynomials that form a rational function, of which the degree of the numerator pn (x) is exactly one more than the degree of the denominator qm (x), then
pn (x) = Q (x) · qm (x) + R     =>      pn (x) / qm (x) = Q (x) + R / qm (x)
 where, Q (x) = ax + b is the quotient and R / qm (x) is the remainder with constant R.
The quotient Q (x) = ax + b represents the equation of the slant asymptote.
As x approaches infinity (or negative infinity), the remainder R/qm(x) vanishes (tends to zero).
Thus, to find the equation of the slant asymptote, perform the long division and discard the remainder.
The graph of a rational function will never cross its vertical asymptote, but may cross its horizontal or slant asymptote. 
The graph of the reciprocal function or equilateral or rectangular hyperbola
The graph of the reciprocal function y = 1/x or  y = k/x is a rectangular (or right) hyperbola of which asymptotes are the coordinate axes.
If k > 0 then, the function is decreasing from zero to negative infinity and from positive infinity to zero, i.e., the graph of the rectangular hyperbola has two branches, in the first and third quadrants as is shown in the right figure. 
The hyperbola has two axes of symmetry.
 The vertices,
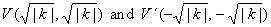Translation of the reciprocal function or linear rational function
The rational function  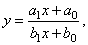by dividing the numerator by denominator,  
 can be rewritten into 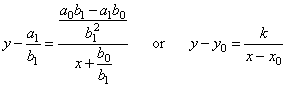where,
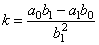is the constant,  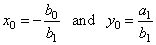are the vertical and the horizontal asymptote respectively.
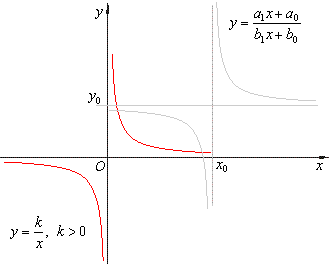
Therefore, the values of the vertical and the horizontal asymptotes correspond to the coordinates of the horizontal and the vertical translation of the reciprocal function  y = k/x as is shown in the figure below.
Graphing rational functions examples
Example:  Given the rational function 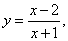sketch its graph.
Solution:  The vertical asymptote can be found by finding the root of the denominator,
                      x + 1 = 0       =>       x =  -1  is the vertical asymptote.
The horizontal asymptote is the ratio of their highest degree term coefficients since the degree of polynomials in the numerator and denominator are equal,
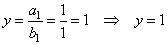  is the horizontal asymptote.
The graph of the given rational function is translated equilateral (or rectangular) hyperbola shown below.
The rational function of the form
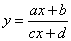can be rewritten into
so  
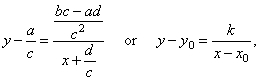where, x0 and y0 are asymptotes and k is constant.
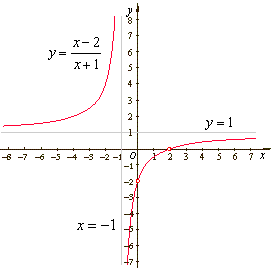Therefore, values of the vertical and the horizontal asymptote correspond to the coordinates of the horizontal and the vertical translation of the source equilateral hyperbola  y = k/x, respectively.
Example:  Given the rational function   sketch its graph.
Solution:  The vertical asymptote can be found by finding the root of the denominator, 
                      x + 2 = 0       =>      x =  -2  is the vertical asymptote.
Since the degree of the numerator is exactly one more than the degree of the denominator the given rational function has the slant asymptote.
By dividing the numerator by the denominator
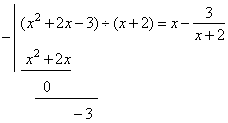obtained is the slant asymptote  y = x and the remainder  3/(x + 2) that vanishes as x approaches positive or negative infinity.
 
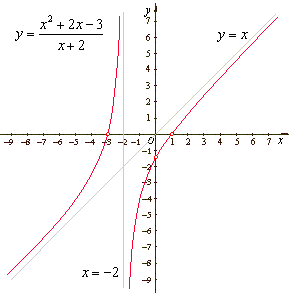Functions contents C
Copyright © 2004 - 2020, Nabla Ltd.  All rights reserved.