|
|
|
Quadratic Equations and Quadratic
Function |
Quadratic function or
the second-degree polynomial |
|
Vertex
(maximum/minimum) - coordinates of translation
|
|
Roots or zeros of the
function, axis of symmetry and y-intercept |
|
Transformations
of the quadratic function's expression |
| |
|
|
|
|
|
| Quadratic function or
the second-degree polynomial |
| The
polynomial function of the second degree, f
(x)
=
a2x2
+ a1x
+ a0, is called a
quadratic function. |
 |
| |
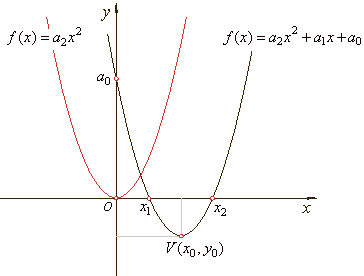y
= f (x)
=
a2x2
+ a1x
+ a0 or
y
-
y0
= a2(x
-
x0)2, |
|
|
| where |
 |
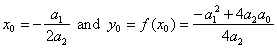are the coordinates
of translations of the quadratic |
|
|
function. By
setting x0
= 0
and
y0
= 0 we obtain
y
=
a2x2,
the source quadratic function.
|
|
The turning point V(x0,
y0)
is called the vertex of the parabola.
|
|
Note that the coefficients, a2, a1
and a0,
of quadratic function, correspond to the coefficients, a, b
and c,
of
quadratic equation, respectively.
|
| The
real zeros of the
quadratic function: |
 |
|
|
|
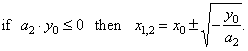The above formula is known quadratic formula
that shows the
symmetry of the roots relative to the axis of symmetry of the
parabola.
|
| |
y
= f (x) =
a2 x2
+ a1x
+ a0 = a2
(x
- x1)(x
- x2)
= a2 [ x2
-
(x1 +
x2)
x
+
x1x2
] |
|
|
| The graph of a quadratic function is curve called a parabola. The parabola is symmetric with respect to a vertical line
called the axis of symmetry. |
| As
the axis of symmetry passes through the vertex of the parabola
its equation is x
= x0. |
| Quadratic
function has the y-intercept at the
point ( 0,
a0 ).
|
|
| Translated form of
quadratic function |
| The
proof that quadratic function f
(x)
= a2x2 + a1x
+ a0
is translation of its source or original
f (x)
= a2x2 |
| 1)
Let calculate the
coordinates of translations of quadratic function using the
formulas, |
| substitute
n
= 2 in |
 |
|
|
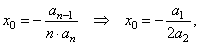| then |
 |
|
|
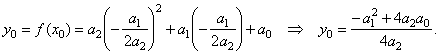| 2)
To
get the source quadratic function we should plug the coordinates
of translations (with changed signs)
into the general form
of the quadratic,
i.e., |
 |
| after
expanding and reducing obtained is
y
=
a2x2
the source quadratic function |
| 3)
Inversely, by plugging the coordinates of translations into the source quadratic function |
|
y
-
y0
= a2(x
-
x0)2, |
|
|
| and
after
expanding and reducing we obtain |
|
y
=
a2x2
+ a1x
+ a0 the quadratic function
in the general form. |
|
|
|
|
|
|
|
|
|
|
| Functions
contents A |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
