Integral calculus
      The indefinite integral
      Integration by parts rule
      The indefinite integrals containing quadratic polynomial (trinomial)
         The indefinite integrals containing quadratic polynomial, examples
Integration by parts rule
The rule for differentiating the product of two differentiable functions leads to the integration by parts formula.
Let  f (x) and g (x) are differentiable functions, then the product rule gives
[ f (x) g (x)]' =  f (x) g (x)'  +  g (x) f ' (x),
by integrating both sides
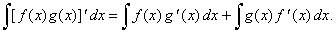
Since the integral of the derivative of a function is the function itself, then
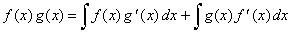
and by rearranging obtained is
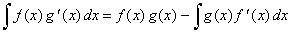
the integration by parts formula.
By substituting  u = f (x) and  v = g (x) then,  du = f ' (x) dx and  dv = g' (x) dx, so that 
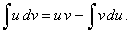
To apply the above formula, the integrand of a given integral should represent the product of one function and the differential of the other.
The selection of the function u and the differential dv should simplify the evaluation of the remaining integral.
In some cases it will be necessary to apply the integration by parts repeatedly to obtain a simpler integral.
  57.  Evaluate
 
Use similar methods to solve this integral as in the preceding example. Hence, the solutions depend on the sign of the leading coefficient a and the sign or the value of the vertical translation y0.
Example:  57 a )  Evaluate
 
Let solve given integral by separating the derivative of the quadratic polynomial in the numerator.
Solution: 
  58.  Evaluate
Use the substitution  mx + n = 1/ t .
Example:  58 a )  Evaluate
Solution: 
  59.  Evaluate
Solution:
The integrand function to be real, the quadratic polynomial must be positive, therefore
 a )   if  a > 0  and   y0 is not 0  then
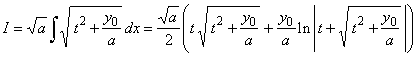
see the solutions of the integrals, example 31 and 36. By using above substitutions 
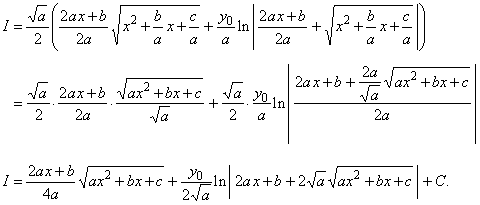
Note that the sign of the vertical translation y0 affects the solution, i.e., changes the sign of its second term.
 b )   if  a < 0  and   y0 > 0  then 
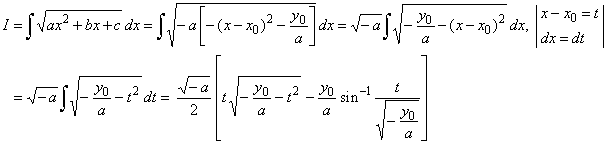
see the solution of the integral example 8 above. After applying the substitutions
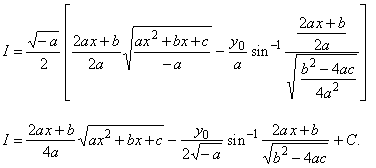
Functions contents G
Copyright © 2004 - 2020, Nabla Ltd.  All rights reserved.