|
|
|
Exponential and Logarithmic
Functions and Equations
|
Rules and properties of logarithms
|
|
Natural logarithm, common logarithm
|
|
Changing the base – different
logarithmic identities
|
|
|
|
|
|
|
| Rules and properties of logarithms
|
| A
logarithm is the exponent (the power) to which a base must be
raised to yield a given number, that is |
| y =
loga
x
if x = a
y. |
| Examples: |
 |
|
|
|
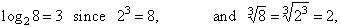| Notation:
Common logarithms of x,
log10 x
(to the base 10) are often written log
x, without the base
explicitly indicated while, Natural
logarithms, loge
x (to the base e,
where e = 2,718218... ), are written ln
x. |
| Therefore, |
|
loga
ax =
x |
 |
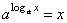loga
a = 1
|
loga 1 = 0
|
|
| Logarithms
are used to simplify multiplication, division and exponentiation
so that, |
| |
loga
(m · n) =
loga
m +
loga
n |
|
 |
|
|
| |
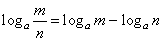loga
mn =
n ·
loga
m
|
|
 |
|
|
|
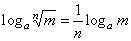| Example:
Using the rules of logarithms find the value of x. |
| Solution: |
 |
|
|
|
|
|
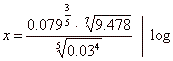| Changing the base – different
logarithmic identities
|
| Using
the identity |
 |
 |
|
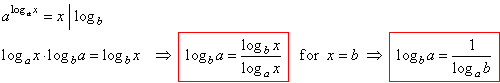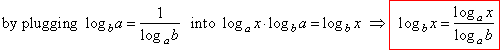| In
a similar way |
|
|
 |
|
 |
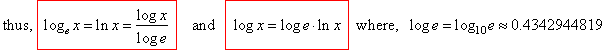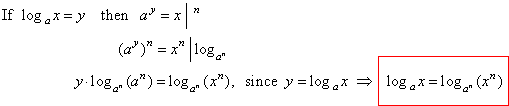| therefore, |
 |
|
|
|
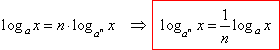| Similarly, |
 |
|
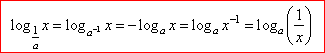| and |
 |
|
|
|
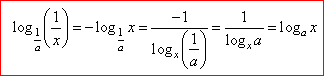| Example:
If log 2
x
= 7 then log
4 2x = ?. |
|
|
|
| Example:
If a
= log 10 2 then
log 10 25
= ?. |
|
|
|
| Example:
Find the value of the expression |
 |
|
|
|
|
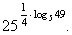| Example:
Find the value of the expression |
 |
|
|
|
|
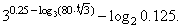| Example:
Find the value of the expression |
 |
|
|
|
|
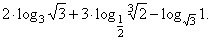| Example:
If a
= log 5 and b = log 3
then log
30 8 = ?. |
|
|
|
|
|
| Example:
Find the value of the expression |
 |
|
|
|
|
|
|
|
|
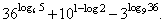