|
|
Power
series |
 Maclaurin and Taylor series
Maclaurin and Taylor series |
 The power series expansion of the exponential
function
The power series expansion of the exponential
function |
|
Properties
of the power series expansion of the exponential function |
|
The radius of convergence or the interval of convergence |
|
|
|
|
|
|
| Maclaurin and Taylor series |
| Consider
the polynomial function |
|
f (x) = an
xn
+ an -
1xn -
1 + · · · + a3x3
+ a2x2 + a1x
+ a0.
|
| If
we write the value of the function and the values of its successive
derivatives, at the origin, then |
| f
(0) =
a0, f '(0) = 1· a1,
f ''(0) = 1· 2a2,
f '''(0) = 1· 2· 3a3, . . . , f (n)(0) =
n!an |
| so we
get the coefficients; |
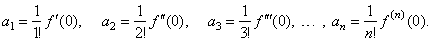 |
|
| Then,
the polynomial
f (x)
with infinitely many terms, written as the power series |
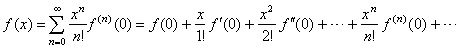 |
and |
|
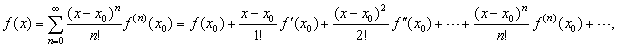 |
| where
0! = 1,
f (0) (x0)
= f
(x0)
and
f (n)
(x0)
is the nth
derivative of f at
x0, |
|
represents an infinitely differentiable function
and is called Maclaurin series and Taylor series respectively. |
|
| The power series expansion of the exponential function |
| Example: Let
represent the exponential function f
(x)
= ex
by the infinite polynomial (power series). |
| Solution: The
exponential function is the infinitely differentiable function defined
for all real numbers whose |
| derivatives
of all orders are equal ex
so that, f (0)
= e0 = 1,
f (n)(0)
= e0 = 1 and |
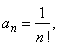 |
therefore |
|
| the function can be represented as a power
series using
the Maclaurin's formula |
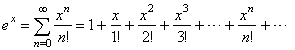 |
| the exponential function
is represented
by the power series that is absolutely convergent for all real x
since |
| by
the ratio test |
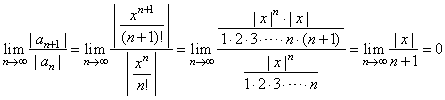 |
|
| the
limit L
< 1 for
any value of x. |
 |
|
| Properties
of the power series expansion of the exponential function |
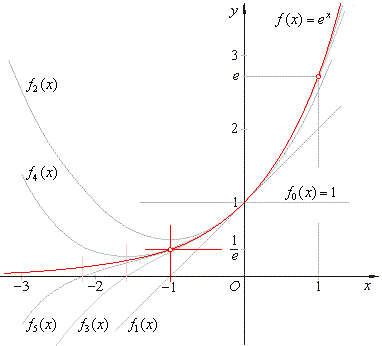
| Since
every polynomial function in the sequence, f1(x),
f2(x),
f3(x),
. . . , fn(x),
represents translation of its
original or source function that passes through the origin, we calculate the coordinates of
translations, x0 and
y0,
to get their source forms. |
| Now
we apply the method and formulas that are revealed and
explored under the 'Polynomial' sections.
Thus, the coordinates of translations, |
|
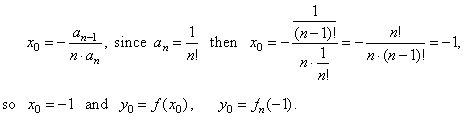 |
| Note
that the above result proves the main property of the polynomial stating
that, an nth
degree polynomial function and all its successive derivatives to the (n
-
1)th order, have constant horizontal translation
x0. |
| Below
listed sequence of the polynomials and corresponding vertical
translations y0
shows that their graphs
approach closer and closer to the point (x0,
y0) or (-1,
1/e) as n
tends to infinity. |
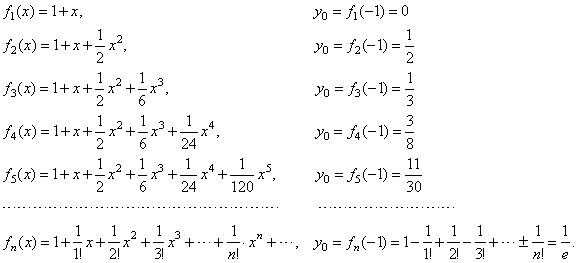 |
| By
plugging the coordinates of translations with changed signs into the
polynomial expressed in the general |
| form
y + y0
= an(x + x0)n
+ an -
1(x + x0)n -
1 + · · · + a2(x +
x0)2 + a1(x + x0)
+ a0, |
| after
expanding and reducing the expression, we get the source polynomial
function passing through the origin.
The above expression we can write as |
| fs(n)(x)
+ y0 = fn(x + x0)
or fs(n)(x)
= fn(x + x0) -
fn(x0), |
| For
example, we obtain the source quadratic fs2(x)
from the expression |
| fs2(x)
+ y0 = f2(x + x0)
or fs2(x)
= f2(x + x0) -
y0 that is, |
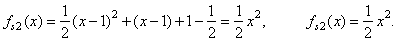 |
| The
same way we get the source function of every polynomial as listed below. |
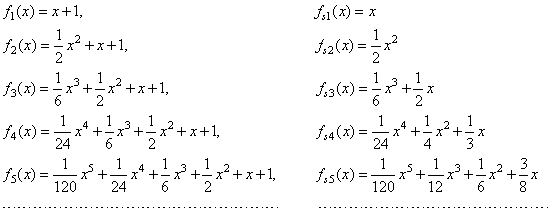 |
| To
prove that expressions on the left and the right side of the same row
represent the same curve plug x0 and
y0
into the source polynomial to get its translated form or, we can check
if the derivative
at x0
= - 1
of the
left side polynomial
is
equal to the derivative at x
= 0 of the source polynomial
(the graph of which passes
through the origin) on the right side. Thus,
for example |
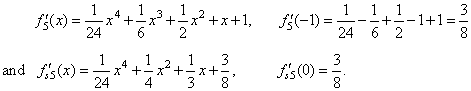 |
| Note
that all polynomials from f1
to fn
in the above sequence have the same horizontal translation x0
= - 1.
|
| Recall
that an nth
degree polynomial function and all its successive derivatives to the (n
-
1)th order, have constant
horizontal translation x0. |
| Since
every polynomial in the above sequence represents the derivative of its
successor, that is, |
| f
'n (x)
= fn -
1(x)
and thus f
'n (x0)
= fn -
1(x0). |
| Therefore
as consequence, each x-intercept
of odd polynomial in the sequence determines the abscissa of the
only extreme (minimum) of succeeding even polynomial and the abscissa of
the only point of inflection of succeeding
odd polynomial, as shows the picture above. |
| In
the same way, for example the coefficients, a1,
a2,
and a3
of the source polynomial fs5(x)
are |
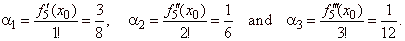 |
| Hence,
the vertical translations y0
of the successive derivatives are, |
|
f4(x0)
= 1! f5´(x0)
= 3/8, f3(x0)
= 2! f5´´(x0)
= 1/3 and
f2(x0)
= 3! f5´´´(x0)
= 1/2 , |
| as
is shown above. |
| Thus,
the power series expansion of the exponential function for x
= 1
yields |
| |
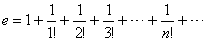 |
from
where we get the number e
as accurate as we please. |
|
| For
x = -1
it yields |
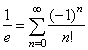 |
and similarly |
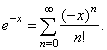 |
|
|
|
| The radius of convergence or the interval of
convergence |
|
- If the power series anxn
converges when x = x1,
then it converges for every x
that is closer to the |
|
origin than x1,
that is, whenever | x
| < | x1 |.
|
|
- If the power series anxn
diverges when x = x1,
then it diverges for every x
that is further from the origin |
|
than x1,
that is, whenever | x
| > | x1 |. |
| The
real power series converges for all absolute value of x
that are less than a number r,
called the radius of convergence or the
interval of convergence,
written | x |
< r that is, the open interval
-
r < x < r. |
| We
apply the root test or the ratio test to find the interval of
convergence. Thus, |
| |
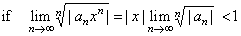 |
the power series |
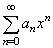 |
converges, |
|
| |
|
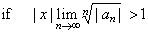 |
the power series diverges. |
|
|
| Therefore
the inequality, |
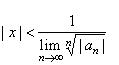 |
|
| defines the interval
in which the power series is absolutely convergent. |
| Thus,
denoting the right side of the above inequality by r,
we get
the interval of convergence
| x | < r
saying, for
every x
between -
r
and r
the series
converges absolutely while, for
every x
outside that interval the series
diverges.
|
| Therefore,
the radius of convergence of the power series, |
 |
| The power series
anxn
converges absolutely at every point x
from the open interval -
r < x < r and
diverges for all
x outside this interval. At
the endpoints -
r
and r,
the series may converge or diverge so, these
points must be checked for convergence, individually. |
|
|
| The power series
an(x
-
x0)n
converges absolutely at every point x
from the open interval |x
-
x0|
< r or |
|
x0
-
r < x < x0
+ r and
diverges for all
x outside this interval.
|
| Note
that the series may or may not converge when | x
-
x0 | = r
that is, when x
=
x0
-
r
or x
=
x0
+
r.
|
| To
determine whether the power series converge or diverge at endpoints we
should plug each endpoint into the
given series and apply appropriate test for convergence. |
|