|
|
|
 Graphing the
polynomial functions
Graphing the
polynomial functions |
|
The
source
or original polynomial function |
|
Translating
(parallel shifting) of the polynomial function |
|
Coordinates of translations
and their role in the polynomial expression |
 Translated
power function
Translated
power function |
|
Translated
sextic function example
|
|
|
|
|
|
|
|
| Graphing the
polynomial functions |
| Translating
(parallel shifting) of the polynomial function |
| Thus,
to obtain the graph of a given polynomial function f
(x)
we translate (parallel shift)
the
graph of its source function in the direction of the x-axis
by x0
and in the direction of the y-axis
by y0. |
| Inversely,
to put a given graph of the polynomial function beck to the
origin, we translate it in the opposite direction, by taking the
values of the
coordinates of translations with opposite sign. |
|
| Coordinates of translations
and their role in the polynomial expression |
| The
coordinates of translations we calculate using the formulas, |
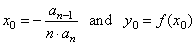
|
| Hence,
by plugging the coordinates of translations into
the source polynomial function fs(x),
i.e., |
|
y
- y0
= an(x
- x0)n
+ an-2(x
- x0)n-2
+
.
. .
+
a2(x
- x0)2
+ a1(x
- x0) |
|
|
| and
by expanding above expression we get the polynomial function in
the general form |
| f
(x) =
y = anxn
+ an-1xn-1
+ an-2xn-2
+
.
. . +
a2x2
+
a1x + a0. |
| Inversely, by plugging the coordinates of translations into
the given polynomial f
(x)
expressed in the general form,
i.e., |
|
y
+ y0
= an(x
+ x0)n
+ an-1(x
+ x0)n-1
+
.
. .
+ a1(x
+ x0)
+ a0 |
|
|
| and
after expanding and reducing above expression we get its source polynomial function. |
| Note
that in the above expression the signs of
the coordinates of translations are already changed. |
|
| Translated
monomial (or
power) function |
| If
we set all coefficients, an-2
to a1,
in the above expanded form of the
polynomial to zero, we get |
| y
- y0
= an(x
- x0)n,
x0
= -
an-1/(
n · an)
and y0
=
f
(x0). |
| the
translated
power (or monomial) function, the exponent of which is an odd or
an even positive integer. |
| When
the exponent is even, i.e., of the form n
= 2m,
m Î N,
the graph of the source power function |
| y
= anxn
is symmetric about the y-axis,
that is f (-x)
=
f (x). |
| When
the exponent is odd, i.e., of the form n
= 2m
+ 1,
m Î N,
the graph of the source power function |
| y
= anxn
is symmetric about the origin, that
is f (-x)
=
-f
(x). |
|
|
|
| Example:
Given is sextic y
= (1/4)x6 -
6x5
+
60x4
-
320x3
+
960x2
-
1536x
+
1008,
find its source or original function and calculate
the coordinates of translations, the zero points and the turning
point. |
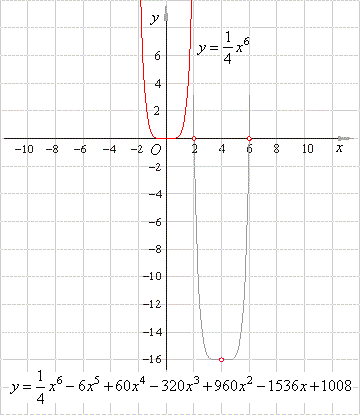
| Draw
graphs of the source and the given sextic. |
| Solution:
1)
Calculate the coordinates of translations |
 |
| y0
= f (4)
= (1/4)
·
46
-
6 ·
45
+
60
·
44
-
320 ·
43
+
960
·
42
-
1536 ·
4
+
1008,
y0
= -
16. |
| 2)
To get the source sextic, plug the coordinates of translations
into the general form of the given sextic, to draw
its graph back to the origin, |
| y
+
y0
= a6(x
+
x0)6
+
a5(x
+
x0)5
+
a4(x
+
x0)4
+
a3(x
+ x0)3
+
a2(x
+ x0)2
+
a1(x
+ x0)
+
a0
or |
| y
-
16 = (1/4)(x
-
4)6 -
6(x
-
4)5
+
60(x
-
4)4 -
320(x
-
4)3
+
960(x
-
4)2
-
1536(x
-
4)
+
1008, |
| after
expanding and reducing above expression obtained is |
| y
= (1/4)
x6 - the
source sextic. |
| Since
all the coefficients, a4,
a3,
a2
and a1,
of the source sextic y
=
a6x6
+ a4x4
+ a3x3
+ a2x2
+ a1x,
are
zero then, the only turning point is T(x0, y0)
or T(
4, -
16). |
| 3)
Inversely, by plugging the coordinates of translations into the source
sextic |
| y
- y0
= a6(x
- x0)6
or
y
- 16
= (1/4)(x -
4)6 |
| what
after expanding yields |
| y
= (1/4)
x6 -
6x5
+
60x4
-
320x3
+
960x2
-
1536x
+
1008 |
| the
given sextic. Therefore, the given sextic is translated monomial or
power function. |
| As
the translated monomial or power function has zeros if a6
· y0
< 0 then. |
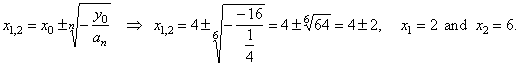 |
 |
|
|
|
|
|
|
|
|
|
|
| Calculus
contents A |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
|
|