Differentiation of an indefinite integral with respect to upper and lower limits, The fundamental theorem of calculus, The fundamental theorem of differential calculus, The fundamental theorem of integral calculus

119

Integral calculus

The indefinite integral

Differentiation of an indefinite integral with respect to upper and lower limits

Let's prove that the differentiation of an indefinite integral with respect to the variable upper limit yields the function that is integrated, the integrand.

Let write the increment DF of the difference quotient DF/Dx

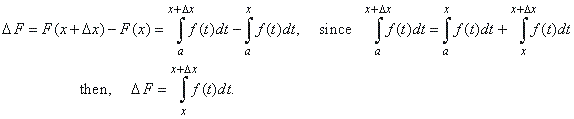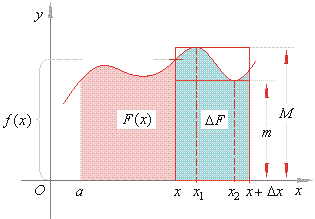
As f (x) is continuous function in the closed interval [x, Dx] it takes its maximal value M and minimal value m at some points, x₁ and x₂, as is shown in the right figure.

Therefore, according to the mean value theorem

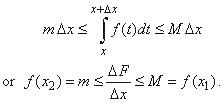Now, as Dx ® 0 then x + Dx tends to x, and since f is continuous then f(x₁) and f(x₂) approach f (x).

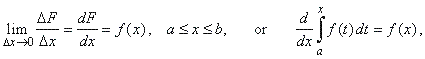that is, by differentiating the indefinite integral of a continuous function with respect to the upper limit x we get the function that is integrated (the integrand). Hence, we also can write

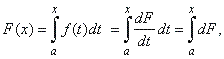that is, by integrating the derivative F' (x), or the differential dF, we get the antiderivative F (x).

Therefore, differentiation and integration are inverse-related operations, when successively performed on the same continuous function, the function stays unchanged. So, by integrating a continuous function f we get a new function which, when differentiated, leads back to the original function f.

This property we use to check the result of integration thus, by differentiating the result of integration we must obtain the integrand (the function that is integrated).

Similarly, differentiation of the indefinite integral with respect to the lower limit we write as

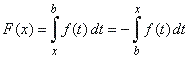so that, F' (x)dx = - f (x).

Therefore, the indefinite integral of a continuous function f (x) is any of its antiderivatives or primitive functions (whose derivative is f (x)).

The fundamental theorem of calculus

The theorem that states the relationship between integration and differentiation, that is, between areas and tangent lines, is called the fundamental theorem of calculus

The fundamental theorem of differential calculus

If f (x) is continuous on closed interval [a, b] and F (x) is defined to be

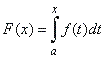then, F is differentiable on (a, b) such that F' (x) = f (x) for all x in (a, b). This means that

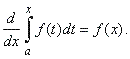Evaluating definite integrals using indefinite integrals

To evaluate the definite integral

we should find one primitive function F (x) or antiderivative

of the function f (x), and since the indefinite integral

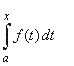is a primitive function of f (x) then,

as two primitives of the same function can differ only by a constant, we can write

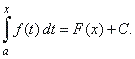To find the value of the constant C that belongs to the lower limit a, substitute x = a to both sides of the

above equality, and since

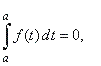then C = - F(a), so that

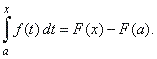Therefore, the definite and indefinite integrals are related by the fundamental theorem of calculus.

This result shows that integration is inverse of differentiation.

The fundamental theorem of integral calculus

If f (x) is integrable on the interval [a, b] and F (x) is an antiderivative of f on (a, b), then

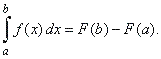The right side of the above equation we usually write

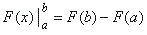so that,

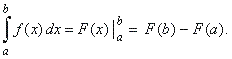Thus, to evaluate the definite integral we need to find an atiderivative F of f, then evaluate F (x) at x = b and at x = a, and calculate the difference F (b) - F (a).

Evaluating the definite integral examples

Example: Find the area under the line f (x) = x + 1 over the interval [1, 5].

Solution: To evaluate the definite integral we need to find the atiderivative F of f (x) = x + 1, evaluate

F (x) at x = 5 and at x = 1, and calculate the difference F (5) - F (1).

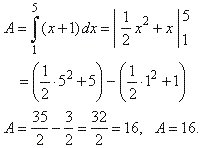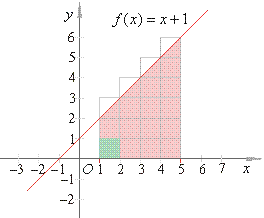Cavalieri - Gregory formula for quadrature of the parabola

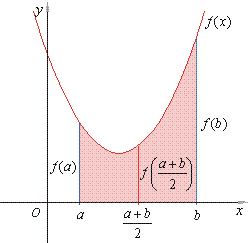
Example: Let define the surface area enclosed by the arc of the parabola f (x) = Ax² + Bx + C and x-axis over the interval [a, b].

Solution:

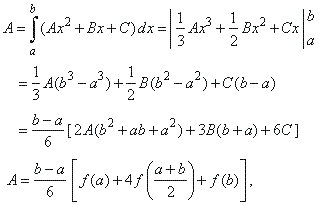where the expression in the square brackets

Thus, the quadrature of the quadratic function leads to calculation of the three ordinates or values of the function, f (a), f ((a+ b)/2) and f (b).

Contents L