|
|
|
|
Trigonometry
|
|
|
|
Trigonometric
equations
|
|
An equation that involves one or more trigonometric functions, of an unknown arc, angle or number, is
called trigonometric equation.
|
|
Basic trigonometric
equations
|
|
The equation sin
x = a, -1
<
a <
1
|
|
To solve the equation we should find the arc x (or angle in radians) of which function value of sine equals a.
Infinite many arcs whose sine value equals
a
end in the points P
and P′
that is,
|
|
x
=
arad
+ k 2p
= arcsin a
+ k 2p,
k Î
Z,
and
|
| x′
= (p
- arad)
+ k 2p
= (p
- arcsin
a)
+ k 2p,
k Î
Z,
|
|
there are an infinite number of solutions obtained by giving different
integer values to
k. This is the set of the
general solutions of the
given trigonometric equation.
|
| For
k = 0 obtained
are, |
| x0
= arcsin a
and x0′
= p - arcsin a, |
|
or x0
= sin-1
a
and x0′
= p - sin-1
a, |
| the
basic
solutions.
|
|
 |
|
|
The principal values of the inverse sine function,
arcsin or
sin-1,
are those between -
p/2 and
p/2. |
|
The above solutions of the equation
sin x =
a, -1
<
a <
1 can concisely be written as
|
| x
= (-1)k
· arcsin a
+ k
·
p,
k Î
Z.
|
| For example,
a) sin x
= -1,
b)
sin
x
=
0,
c)
sin
x
= 1, |
| x
= -
p/2
+ k 2p.
x
= k
p.
x
= p/2
+ k 2p,
k Î
Z.
|
| Using a scientific calculator to obtain principal (or basic) value of the inverse sine function, arcsine, input value
for
a
and press, sin-1
(or INV sin or 2nd sin).
|
| Obtained is an arc (in radians) or angle (in degrees)
between -
p/2 and
p/2, depending on what measurement (DEG or
RAD) was set initially, by the DRG key.
|
|
|
Example:
Solve
the equation, |
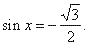 |
|
|
Solution:
Let
first find basic solutions of the equation.
We should remember values of the trigonometric
|
| functions of some special arcs (angles) like
|
 |
|
|
Since the basic solutions
for the equation sin x =
a are,
x0
=
arad
and x0′
= p -
arad,
then
|
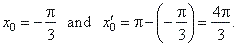 |
| While from
x
= x0 + k
·
2p
and x′
= x0′
+ k
·
2p,
k Î
Z,
follow
|
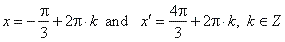 |
the general solutions
of the given equation.
|
|
|
The basic solutions we see on the unit circle in
the below figure while the graph shows that the line
y = -
Ö3/2
intersects the sine function at infinite many points whose abscissas represent the general solutions.
|
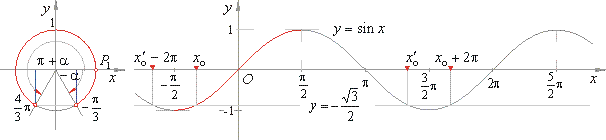 |
|
|
The equation
cos
x = a,
-1
<
a <
1
|
|
The solutions of the equation are arcs
x
whose function's value
of cosine equals a.
|
|
Infinite many arcs whose cosine value equals a end in points,
P
and P′, that are |
| x
=
arad
+ k 2p
and x′
= - arad
+ k 2p,
k Î
Z.
|
|
This is the set of the general solutions of the given equation.
|
| For
k = 0 follows the basic solutions of the equation |
| x0
=
arad
and x0′
= - arad. |
|
 |
|
|
Therefore, if cos
x = a,
-1
< a <
1
then,
x = +
arad
+ k 2p
= + arccos a, k Î
Z.
|
|
For example if, a =
-1,
then, cos
x = -1
=> x = p
+ k 2p,
k Î
Z,
|
|
a = 0
cos x =
0 =>
x = p/2
+ k p,
k Î
Z, |
|
or
a =
1
cos x =
1 =>
x = k 2p,
k Î
Z.
|
| Since cosine function passes through all values from range
-1
to 1
while arc x
increases from 0
to p, one of the arcs from this interval must satisfy the equation
cos x = a. This arc, denoted
x0,
we call the
basic solution.
|
|
Thus, the basic solution of the equation
cos x = a,
-1
< a <
1
is the value of inverse cosine function,
|
| x0
= arccos a or
x0
= cos-1
a, |
| that is, an arc or angle (whose cosine equals
a) between
0
and p
which is called the principal
value.
|
|
Scientific calculators are equipped with the
arccos (or
cos-1) function which, for a given argument between
-1
and 1, outputs arc (in radians) or angle (in degrees) from the range
x0 Î
[0,
p].
|
|
|
Example:
Solve
the equation, cos
x = -
0.5.
|
|
Solution:
In the unit circle in
the below figure shown are the two arcs, of which cosine value equals
-
0.5, that represent the basic solutions of the given equation
|
| x0
= 120°
or
x0′ =
-
120°
|
|
while the abscissas of the intersection points of the line
y = -
0.5 with the graph of cosine function represent
the set of the general solution
|
|
x
= +
120°
+ k
·
360°
or
x
= +
2p/3
+ k 2p,
k Î
Z.
|
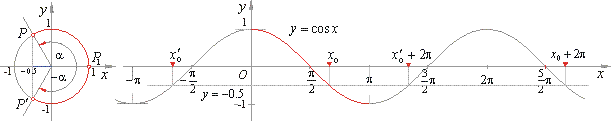 |
| The same results we obtain by using calculator if we set DEG then input
|
| -
0.5 INV
cos
(or cos-1)
=> x0
= 120°
and
x0′ =
-120° that
are the basic solutions.
|
| Or we input the same while calculator is set in RAD mode to get the arc in radians that is
|
| x0
= 2.094395102rad
= 2p/3rad.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Contents
E
|
|
|
|
|

|
|
|
|
|
|
Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved.
|
|
|