|
Applications
of trigonometry
|
|
Mollweide's formulas, the tangent
law and half-angle formulas - Oblique or
scalene triangle
|
|
|
|
Oblique or
scalene triangle - The tangent
law (or the tangent rule)
|
|
Dividing appropriate pairs of Mollweide's formulas and applying following identities,
|
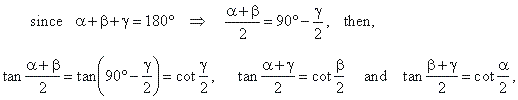 |
|
obtained are equations that represent the tangent law |
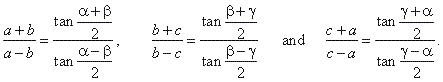 |
|
|
Oblique or
scalene triangle
- Half-angle formulas
|
|
Using the cosine law, appropriate
identities and denoting a
+ b + c = 2s,
|
 |
|
when plugged into the above formula, gives
|
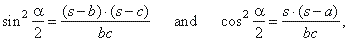 |
|
dividing above expressions obtained are |
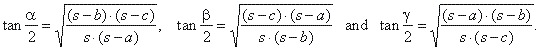 |
|
|
Area of a triangle, the radius of
the circumscribed circle and the radius of the inscribed circle
|
|
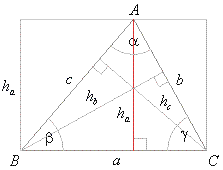
Rectangular in the
figure below is composed of two pairs of congruent right triangles
formed by the given oblique triangle. Therefore, the area of a triangle equals the half of the rectangular
area,
|
 |
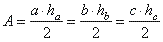 |
|
In the right triangles in the
left figure,
|
| ha
= b · sing,
hb
= c · sina,
hc
= a · sinb, |
|
and
by plugging into above formulas for the area
|
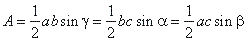 |
|
|
|
the
area of a triangle in terms of an angle and the
sides adjacent to it.
|
|
If, in the above formulas for the area, we substitute each side applying the
sine law, that is
|
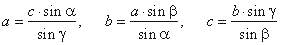 |
|
obtained is
the area of a triangle in terms of a side and all its
angles,
|
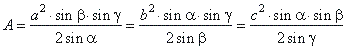 |
|
|
The radius of the circumscribed circle or circumcircle
|
|
Using known relation, which states that the angle subtended by a chord at the circumference is half the
angle
subtended at the center, from the right triangle in
the below diagram follows,
|
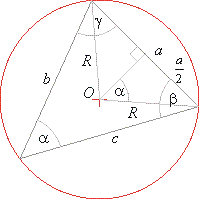 |
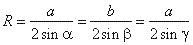 |
|
the radius of the circumscribed
circle,
or
|
| a
= 2R · sina,
b
= 2R · sinb,
c
= 2R · sing. |
|
Plugging the sides into
A
= (1/2) ab sing
obtained is
|
| A
= 2R2 · sina
· sinb
·
sing |
|
|
|
the
area of a triangle in terms of the radius of circumcircle and
all its angles.
|
|
|
Area of a triangle in terms of the inscribed circle (or incircle) radius
|
|
The oblique triangle
ABC in
the figure below consists of three triangles, ABO,
BCO
and ACO
with the same
altitude r
therefore, its area can be written as
|
 |
 |
| where |
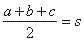 |
is the semi-perimeter
then |
|
|
A
= r
· s is the
area of a triangle |
|
in terms of the semi-perimeter and
inradius. |
|
|
|
The parts of a triangle denoted as in the diagram relates as
follows,
|
| xA
+ xB
= c,
xA
+ xC
= b,
xB + xC
= a
then, 2xA
= -
(xB + xC) + b + c =
-
a + b + c, |
| 2xB
= a -
(xA + xC) + c =
a -
b + c and
2xC
= a + b -
(xA
+ xB) + b + c = a + b
-
c, |
|
again
using a
+ b + c = 2s
it follows that xA
= s -
a,
xB
= s -
b and
xC
= s -
c.
|
|
Then, from the right triangles in the diagram,
|
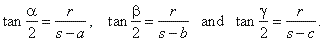 |
|
Equating obtained formulas with the half-angle formulas, as for example
|
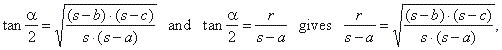 |
|
or |
 |
the radius of the inscribed circle. |
|
|
Plugging given r
into the formula for the area of a triangle A
= r
· s
yields
|
|
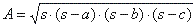 |
Heron's formula. |
|
|
|
Oblique or scalene triangle
examples
|
|
Example:
Determine length of sides, angles and area of a triangle of which
a + b
= 17 cm, c
= 15 cm and
angle g =113°.
|
|
Solution: Using Mollweide's formula
|
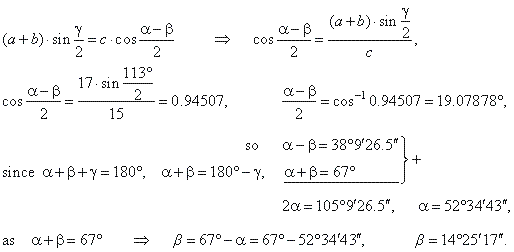 |
|
Applying the sine law,
|
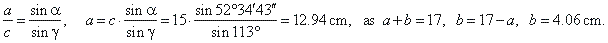 |
| Area of the triangle from the formula |
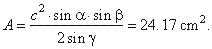 |
|
|
|
Example:
Given is the sum of the sides of a triangle a + b +
c = 46 cm, the radius of the incircle
(or inradius) r =
Ö3
cm and angle
b
=60°. Find all sides and angles of the triangle.
|
|
Solution: Using
the formula |
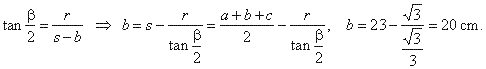 |
| Using Mollweide's formula |
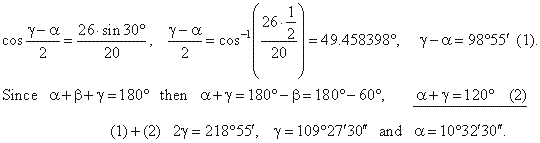 |
| Applying the sine law, |
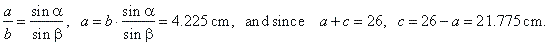 |
|
|
Example:
Determine the area of an isosceles triangle of which, the line segment that joints the midpoint of
one of its equal sides by the midpoint of the base equals the half of the radius
R of the
circumcircle.
|
|
|