|
|
Vectors in a Plane and Space |
|
|
|
|
 Vectors in
three-dimensional space in terms of Cartesian coordinates
Vectors in
three-dimensional space in terms of Cartesian coordinates |
|
Vectors in
a three-dimensional coordinate system examples |
|
|
|
|
|
|
|
Vectors in
a three-dimensional coordinate system examples |
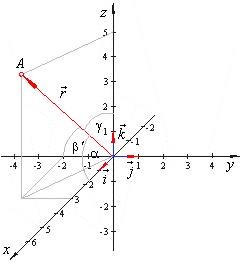
| Example:
Determine angles that a radius vector of the point
A(3,
-2, 5) forms with the coordinate axes. |
| Solution:
Let calculate the magnitude or length of the radius vector, |
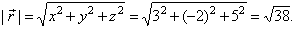 |
| Angles between the radius vector and the coordinate axes are, |
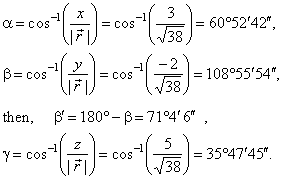 |
|
 |
|
|
| Example:
A vector AB is directed from point
A(-1,
-2,
1) to point B(-2,
3, 4), find the unit vector of the
vector AB. |
| Solution:
Determine the vector AB
from the expression |
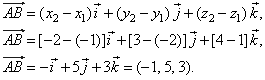 |
| The length of the vector
AB |
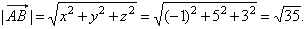 |
| The unit vector of the vector
AB |
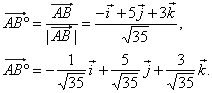 |
|
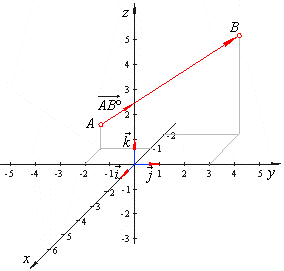 |
|
| Check
that the directional cosines of the unit vector satisfy the relation, |
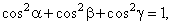 |
|
|
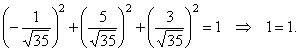 |
|
|
Example: A vector
a
in a 3D-space, of the length | a
|
= 4, forms with axes,
x and
y
the same angles, a
= b
= 60°, find the
components (coordinates) of the vector a. |
| Solution:
Using relation |
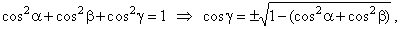 |
|
|
| applying given conditions, |
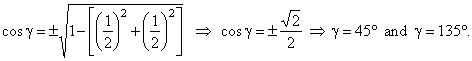 |
|
|
|
|
| Example: Show that vectors,
a
= -i
+ 3 j
+ k,
b
= 3i -
4 j -
2k
and c
= 5i -
10 j -
4k
are coplanar. |
| Solution:
If all three vectors lie on the same plane then there are coefficients,
l and
m such that, for example
c
= la
+ mb,
i.e., each of the vectors can be expressed as the linear combination of the remaining two. |
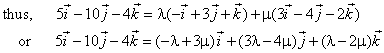 |
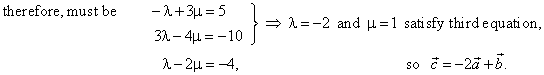 |
|
| Example: Points,
A(0,
-2,
1), B(-2,
1, -3) and
C(3,
-1,
2) are the vertices of a triangle, determine the
vector of the median mc
= CM
and its length. |
| Solution:
The radius vector of the midpoint of the
side AB, |
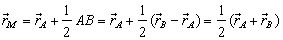 |
|
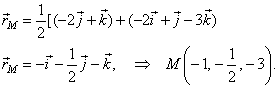 |
| The vector of the median
CM,
mc
= CM
= rm -
rc, |
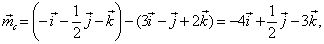 |
| and the length of the median
CM,
|
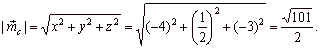 |
|
 |
|
| To check over the obtained result, calculate the coordinates of the centroid
G, |
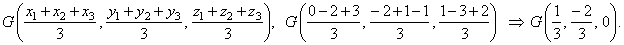 |
| The centroid divides every median in the ratio
2 : 1, counting from the vertex to the midpoint, therefore |
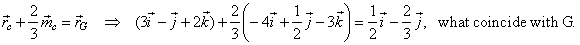 |
|
|
|
|
|
|
|
|
|
|
| Vectors
in 2D and 3D Contents |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
|
|