Unit of measurement of angles, Radian as the circular measure, Protractor an instrument for measuring angles, Degrees to radians and radians to degrees conversion examples, The unit circle or the trigonometric circle, Division of the circumference of the unit circle to the characteristic angles

Trigonometry

Trigonometric Functions

Unit of measurement of angles - a radian (the circular measure)

Protractor - an instrument for measuring angles

Degrees to radians and radians to degrees conversion examples

The unit circle or the trigonometric circle

Division of the circumference of the unit circle to the characteristic angles

Unit of measurement of angles - a radian (the circular measure)

The relation between a central angle a (the angle between two radii) and the corresponding arc l in the circle of radius r is shown by the proportion,

a° : 360° = l : 2rp

It shows that the central angle a° compared to the round angle of 360°

(called perigon) is in the same relation as the corresponding arc l

compared to the circumference 2rp. Therefore,

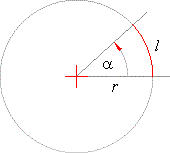
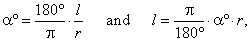where the ratio

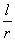we call the circular measure, usually denoted a^rad, i.e.,

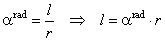thus,

The central angle subtended by the arc equal in length to the radius, i.e. l = r,

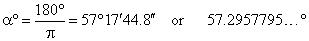we call it radian.

Thus, the angle a = 1° equals in radians,

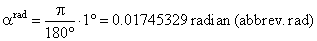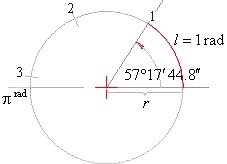or arc1° = 0.01745329. Arc is abbreviation from Latin arcus, (p = 3.1415926535...).

Protractor - an instrument for measuring angles

Mentioned relations between units of measurement of an angle and arc clearly shows the protractor shown in the below figure marked with radial lines indicating degrees, radians and rarely used gradians (the angle of an entire circle or round angle is 400 gradians).

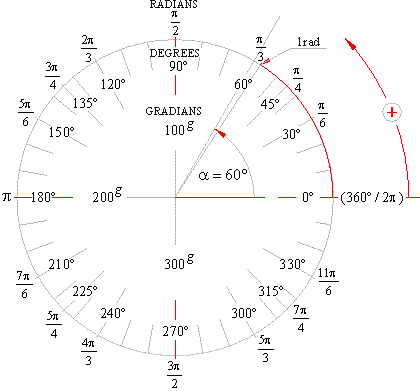A right angle equals 100 grad (gradians).

The hundredth part of a right angle is 1^g grad, and one 100th part of 1grad is centesimal arc minute 1^c, and one 100th part of centesimal arc minute is centesimal arc second 1^cc, therefore

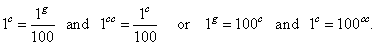Degrees to radians and radians to degrees conversion examples

Example: Convert 67° 18´ 45" to radians.

Solution: The given angle we write in the expanded notation and calculate its decimal equivalent,

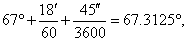then use the formula to convert degrees to radians

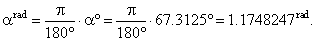Using a scientific calculator, the given conversion can be performed almost direct.

Before a calculation choose right angular measurement (DEG, RAD, GRAD) by pressing DRG key, then input, 67.1845 INV ®DEG 67.3125°

Because a calculator must use degrees divided into its decimal part one should press ®DEG (or ®DD) to get decimal degrees. Then press INV DRG® to get radians, 1.174824753^rad.

Example: Convert 2.785^rad to degrees, minutes and seconds.

Solution: Using formula,

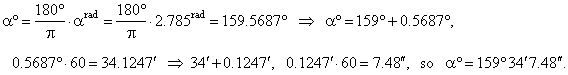The same result one obtains with a calculator through the procedure, press DRG key to set RAD measurement, then input 2.785 INV DRG® 177.2986066 grad = 177^g29^c86^cc, press again INV DRG® 159.5687459° obtained are decimal degrees (DEG), and to convert to degrees/minutes/seconds press INV ®DMS to get 159° 34´ 7.48".

Example: Find the length of the arc l that subtends the central angle a = 123° 38´ 27" in the circle of radius r = 15 cm.

Solution: First express the angle a in decimal degrees, i.e.

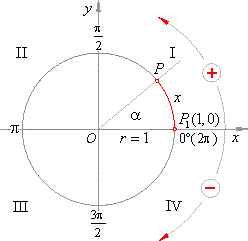
The unit circle or trigonometric circle

A circle of radius r = 1, with the center at the origin O(0, 0) of a coordinate system, we call the unit or trigonometric circle, see the figure below.

The arc of the unit circle that describes a point traveling anticlockwise (by convention, clockwise is taken to be negative direction) from the initial position P₁(1, 0) on the x-axis, along the circumference, to the terminal position P equals the angular measure/distance x = a^rad, in radians.

An angle is in standard position if its initial side lies along the

positive x-axis.

If we take the positive direction of the x-axis as the beginning

of a measurement of an angle (i.e., a = 0^rad, both sides of

an angle lie on the x-axis), and the unit point P₁ as the initial

point of measuring the arc, then the terminal side of an angle,

which passes through the terminal point P of the arc,

rotating around the origin (in any direction) describes different

angles, and the terminal point P corresponding arcs,

x = a^rad + k · 2p, k = 0, ±1, ±2, ±3, . . . .

or x = a° + k · 360°, k Î Z.

It means that every arc x ends in the same point P in which ends the corresponding arc a.

Thus, at any point P on the circumference of the unit circle end infinite arcs x = a + k · 2p, which differ by the multiplier 2p, and any number x associates only one point P.

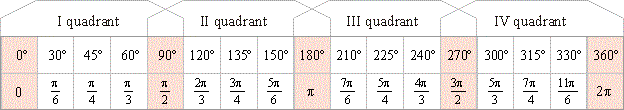
Division of the circumference of the unit circle to the characteristic angles

There is a common division of the circumference of the unit circle to the characteristic angles or the corresponding arcs which are the multipliers of the angles, 30° (p/ 6) and 45° (p/ 4).

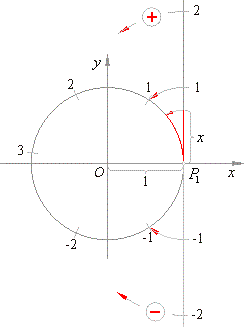
We can say that a unit circle is at the same time

numerical circle.

The numerical circle shown in the right figure is formed

by winding the positive part of number line, with the unit

that equals the radius, around the unit circle in the

anticlockwise direction and its negative part in clockwise

direction.

Therefore, the terms angle, arc and number in the

trigonometric definitions and expressions are mutually

interchangeable.

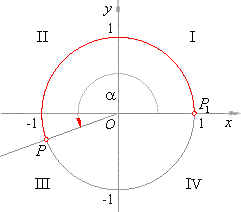
Example: In which quadrant lies second or the terminal side of the angle x = 1280°.

Solution: Dividing the given angle by 360° we calculate the

number of rotations, or round angles, described by terminal side

of the angle x, and the remaining angle a° position of which we

want to find.

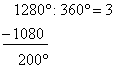since x = a° + k · 360° then k = 3 and a = 200°.

therefore, terminal side of the angle x lies in the third quadrant.

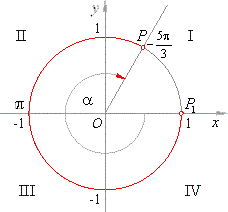
Example: In which quadrant lies the endpoint of the arc x = - 47p/3 of a unit circle.

Solution: Given arc

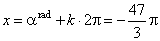can be expanded to

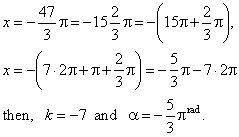Thus, the endpoint of the arc x moved around a unit circle in the clockwise (negative) direction 7 times and described additional arc a = - (5/3)p^rad, so its endpoint P lies in the first quadrant.

Trigonometry contents A