|
| Conic
Sections |
|
|
|
|
Hyperbola
|
Translated hyperbola
|
|
Equation of the hyperbola in vertex form
|
|
Parametric equations of the hyperbola
|
|
Examples of hyperbola |
|
|
|
|
|
|
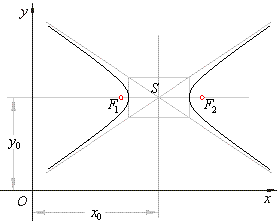
| Translated hyperbola
|
| The equation of a hyperbola translated from standard position so that its center is at
S(x0, y0)
is given by
|
| b2(x
-
x0)2
-
a2(y
-
y0)2
= a2b2 |
| or
|
 |
|
|
|
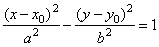and after expanding and substituting constants
obtained is
|
| Ax2
+ By2
+ Cx + Dy + F
= 0.
|
|
An equation of that form represents the hyperbola if
|
|
A · B < 0
|
|
that is, if coefficients of the square terms have
different signs.
|
|
 |
|
|
| Equation of the hyperbola in vertex form
|
By translating the hyperbola, centered at
(0, 0), in the
negative direction of the x-axis by
x0
= -a, so that new
position of the center S(-a, 0)
then its equation is |
|
b2(x
+ a)2
-
a2y2
= a2b2.
After squaring and reducing,
|
| b2x2
+ 2ab2x
-
a2y2
= 0 or |
 |
|
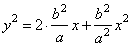| since |
 |
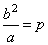obtained is |
 |
|
|
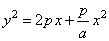the equation of the hyperbola in vertex form.
|
|
 |
|
|
| Parametric equation of the hyperbola
|
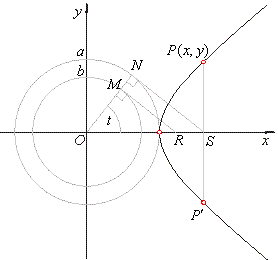
| In the construction of the hyperbola, shown in
the below figure, circles of radii
a
and b
are intersected by an arbitrary line through the origin at points M
and N. Tangents to the circles at
M
and N
intersect the x-axis at
R
and S.
On the perpendicular through S, to the
x-axis, mark the line segment
SP of length
MR to get the point
P of the hyperbola.
We can prove that P is a point of the hyperbola.
|
|
In the right
triangles ONS and
OMR,
|
|
|
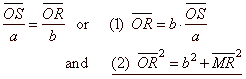
by replacing OS
= x
and MR
= SP
|
|
and substituting |
 |
|
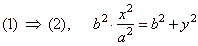| by
dividing by b2, |
 |
|
|
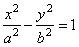therefore, P(x, y)
is
the point of the hyperbola.
|
|
 |
|
| The coordinates of the point
P(x, y)
can also be expressed by the angle t
common to both mentioned |
| triangles,
so that |
 |
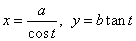is the parametric equation of the hyperbola. |
|
| By substituting these parametrically expressed coordinates into equation of the hyperbola |
 |
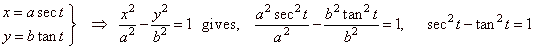| that
is, |
 |
known
trigonometric identity. |
|
|
| Examples
of hyperbola |
Example:
Given is the hyperbola
4x2 -
9y2 = 36,
determine the semi-axes, equations of the asymptotes,
coordinates of foci, the eccentricity and the semi-latus rectum.
|
Solution:
Put the equation in the standard form to
determine the semi-axes, thus
|
|
4x2
-
9y2 = 36 | ¸
36
|
|
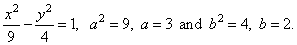
| Asymptotes, |
 |
|
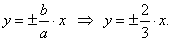| Applying, |
 |
|
|
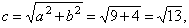coordinates of foci, F1(-Ö13,
0) and
F2(Ö13,
0).
|
|
 |
|
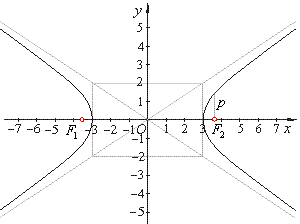| The eccentricity, |
 |
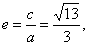and the
semi-latus rectum,
|
 |
|
|
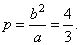| Example:
Write equation of a hyperbola with the focus at
F2(5,
0) and whose asymptotes are, |
 |
|
|
|
 |
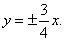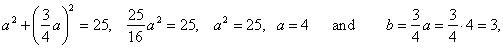| Therefore,
the equation of the hyperbola,
|
 |
|
|
|
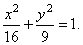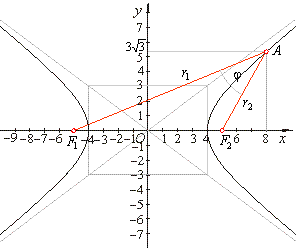
| Example:
Find the angle subtended by the focal radii
r1
and
r2
at a point A(8, y
> 0) of the hyperbola
|
| 9x2
-
16y2 = 144. |
|
Solution:
We determine the ordinate of the point
A
by plugging its abscissa into equation of the hyperbola,
|
|
x
= 8 =>
9x2
-
16y2 = 144
|
|
9 · 82
-
16y2 = 144,
|
|
16y2 = 432 =>
y2 =
27,
|
|
y1,2 = ±3Ö3
so that A(8,
3Ö3).
|
|
The equations of the lines of the radii r1
and
r2, we
write
using the formula of a line through two
points. Since,
|
| r1::
AF1 and
F1(-c,
0) ,
and |
 |
|
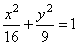| then |
 |
|
|
 |
|
 |
 |
|
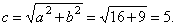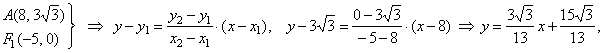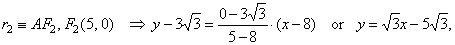Therefore, the angle between the focal radii r1
and
r2
at the point A
of the hyperbola, as
|
 |
|
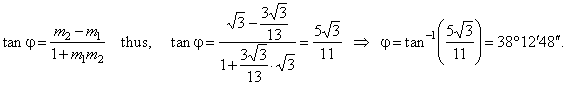| Example:
The hyperbola is given by equation
4x2
-
9y2 + 32x + 54y -
53 =
0. |
| Find coordinates of the
center, the foci, the eccentricity and the asymptotes of the hyperbola. |
| Solution:
The given hyperbola is translated in the direction of the coordinate axes so the values of
translations x0 and
y0
we can find by using the method of completing the square
rewriting the equation in |
| the standard
form, |
 |
|
|
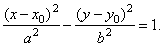Thus,
4x2 + 32x
-
9y2 + 54y -
53 =
0,
|
|
4(x2 + 8x) -
9(y2 -
6y) -
53 =
0
|
|
4[(x +
4)2 -
16] -
9[(y -
3)2 -
9] -
53 =
0
|
|
4(x + 4)2 -
9(y -
3)2 = 36 | ¸
36
|
| Therefore,
|
 |
|
|
 |
|
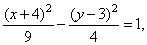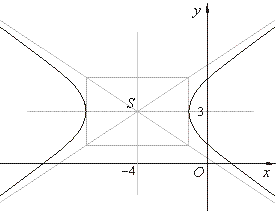| it
follows that a2 =
9, a
= 3,
b2 = 4,
b
= 2,
and the center of the hyperbola at S(x0, y0)
or S(-4,
3). |
| Half
the focal distance |
 |
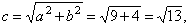the
eccentricity |
 |
|
|
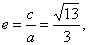and the foci, F1(x0
-
c,
0) so
F1(-4
-
Ö13,
0) and
F2(x0
+ c,
0),
F1(-4
+ Ö13,
0). |
| Equations of the asymptotes of a translated hyperbola |
 |
| therefore, the asymptotes of the given
hyperbola, |
 |
|
|
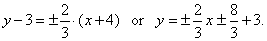| Example:
Write the equation of the hyperbola 9x2
-
25y2 = 225
in the vertex form. |
| Solution:
Using parallel shifting we should place the center of the hyperbola at
S(-a,
0). |
| Rewrite
9x2
-
25y2 = 225 | ¸
225 |
|
 |
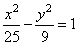therefore,
a = 5 and
b = 3,
so that S(-5,
0). |
|
|
Then, the translated
hyperbola with the center at S(-5,
0) has the equation
|
 |
|
|
|
|
|
|
|
|
|
|
| Pre-calculus contents
H |
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
