|
|
|
|
Ellipse
and Line
|
 Angle
between the focal radii at a point of the ellipse
Angle
between the focal radii at a point of the ellipse
|
 Tangents to an ellipse from a
point outside the ellipse - use of the tangency condition
Tangents to an ellipse from a
point outside the ellipse - use of the tangency condition
|
|
Construction of tangents from a point outside the ellipse
|
 Ellipse and line examples
Ellipse and line examples
|
|
|
|
|
|
|
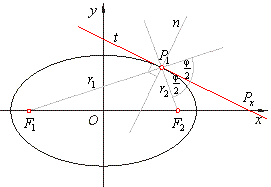
| Angle between the focal radii at a point of the ellipse
|
| Let
prove that the tangent at a point
P1
of the ellipse
is perpendicular to the bisector of the angle between the focal
radii
r1
and r2.
|
|
Coordinates of points,
F1(-c,
0),
F2(c,
0) and
P1(x1,
y1)
plugged into the equation of the line through two given
points determine the lines of the focal radii
|
| r1 =
F1P1
and r2 =
F2P1, |
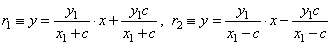 |
|
and the equation of the tangent at the point
P1,
|
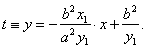
|
|
 |
|
By plugging the slopes of these tree lines into the formula for calculating the angle between lines we find the
exterior angles
j1
and j2
subtended by these lines at P1. |
| Thus, using the condition
b2x12
+ a2y12
= a2b2, that the point lies on the ellipse, obtained is |
 |
| If on the same way we calculate the interior
angle subtended by the focal radii at P1,
and which is the supplementary angle of the angle j, |
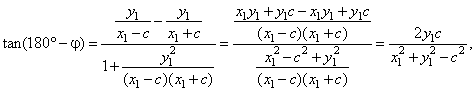 |
| then compare with the
result which will we obtain by using the double-angle formula for the angles
j1
and j2, |
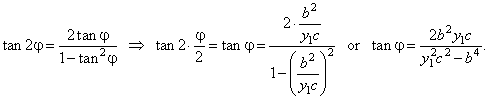 |
| To compare obtained results, we multiply both the
numerator and the denominator of the result for
the supplementary angle by
b2, |
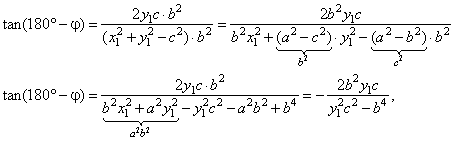 |
| what proved the previous statement. |
| Therefore, the normal at the point
P1
of the ellipse bisects the interior angle between its focal radii. |
|
| Tangents to an ellipse from a
point outside the ellipse - use of the tangency condition
|
| Coordinates of the point
A(x, y), from which we draw tangents to an ellipse, must satisfy
equations of the tangents, y
= mx + c and their slopes and intercepts,
m and
c, must satisfy the
condition of tangency therefore, using the system of equations, |
|
(1) y = mx + c
<=
A(x, y) |
|
(2) a2m2 + b2
= c2
determined are equations of the tangents from a point A(x, y)
outside the ellipse. |
|
|
| Construction of tangents from a point outside the ellipse
|
| With
A
as center, draw an arc through F2, and from
F1
as center, draw an arc of the radius 2a. Tangents are |
|
then the perpendicular bisectors of the line segments,
F2S1 and
F2S2.
|
|
We can also draw tangents as lines through A
and the intersection points of the segments F1S1 and
F1S2
and the ellipse. |
| Thus, these intersections are the tangency points
of the tangents to the ellipse. |
| Explanation of the construction lies at the fact that, |
|
F1S1 =
F1D1 +
D1S1 =
F1D1 +
D1F2 = 2a
|
|
according to the definition of the ellipse, as well as
the point A
is equidistant from points F2 and
S1,
since the point S1
lies on the arc drawn from A
through F2.
|
|
 |
|
|
| Ellipse and line examples
|
| Example:
At a point A(-c,
y
> 0) where c
denotes the focal distance,
on the ellipse
16x2
+ 25y2 = 1600
drawn is a tangent to the ellipse, find the area of the triangle that tangent forms by the coordinate axes. |
| Solution:
Rewrite the
equation of the ellipse to the standard form 16x2
+ 25y2 = 1600 | ¸
1600 |
|
|
| We calculate the ordinate of the point
A
by plugging the abscissa into equation of the ellipse
|
| x =
-6
=>
16x2
+ 25y2 = 1600, |
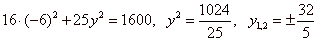
|
|
or, as we know
that the point with the abscise
x = -
c
has the ordinate equal to the value of the
semi-latus rectum,
|
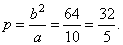
|
|
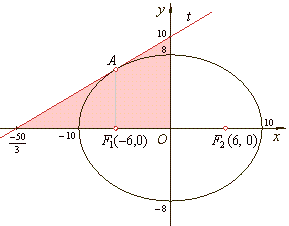 |
|
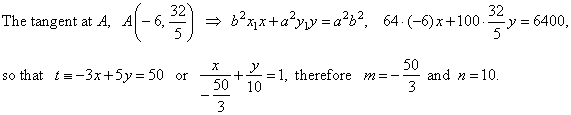 |
| The
area of the triangle formed by the tangent and the coordinate axes, |
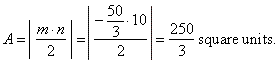 |
|
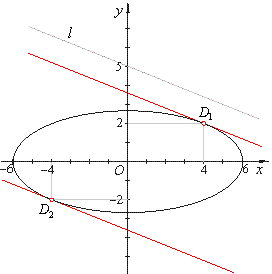
| Example:
Find a point on the ellipse
x2
+ 5y2 = 36 which is the closest, and which is the farthest from the
line 6x + 5y - 25 =
0. |
Solution:
The tangency
points of tangents to the ellipse which are parallel with the given
line are, the
closest and the farthest points from the line.
|
| Rewrite
the equation of the ellipse to determine its axes, |
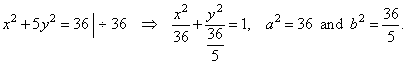 |
| Tangents and given line have the same slope, so |
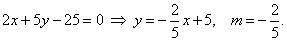 |
| Using the tangency condition, determine the intercepts
c, |
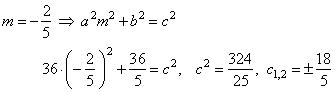 |
|
therefore, the
equations of tangents,
|
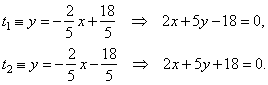
|
|
 |
|
Solutions of the system of equations of tangents to the ellipse determine the points of contact, i.e., the
closest and the farthest point of the ellipse from the given line, thus |
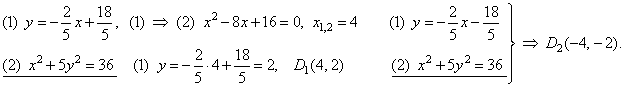 |
|
| Example: Determine equation of the ellipse which the line
-3x +
10y = 25 touches at the point
P(-3,
8/5). |
| Solution:
As the given line is the tangent to the ellipse,
parameters, m
and c
of the line must satisfy the tangency condition, and the point P
must satisfy the equations of the line and the ellipse, thus |
 |
|
| Example:
At which points
curves, x2
+ y2 = 8 and
x2
+ 8y2 = 36, intersect? Find the angle between two
curves. |
| Solution:
Given curves are the circle and the
ellipse. The solutions
of the system of their equations determine the intersection points, so |
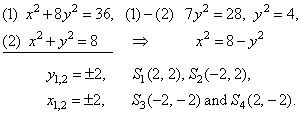 |
|
Angle between two curves is the angle between
tangents drawn
to the curves at their point of
intersection.
|
|
The tangent to the circle at the intersection
S1,
|
|
S1(2,
2) =>
x1x
+ y1y =
r2,
2x +
2y = 8
|
|
or tc
::
y =
-
x
+ 4
therefore, mc
= -1.
|
|
The tangent to the
ellipse at the intersection
S1,
|
|
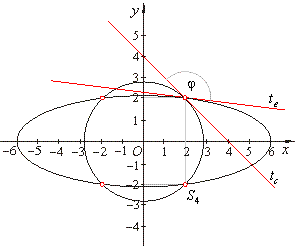 |
|
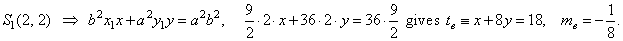 |
| The angle between the circle and the ellipse,
|
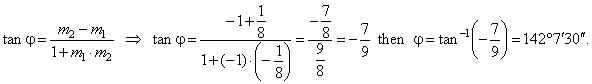 |
|
| Example:
Find the equations of the common tangents of the curves
4x2
+ 9y2 = 36 and
x2
+ y2 = 5. |
| Solution: The common tangents of the ellipse and the circle
must satisfy the tangency conditions of these curves, thus |
|
|
 |
|
|
|
|
|
|