|
|
|
|
|
| The
graphs
of algebraic
and transcendental functions |
| Elementary
functions are, Algebraic functions
and Transcendental functions |
| Algebraic
functions |
|
· The
polynomial function
f (x) =
y = anxn
+ an-1xn-1
+ an-2xn-2
+
.
. . +
a2x2
+
a1x + a0 |
|
y
=
a1x
+ a0
- Linear
function |
|
y
=
a2x2
+
a1x + a0
-
Quadratic
function |
|
y
=
a3x3
+
a2x2
+
a1x + a0
-
Cubic
function |
|
y
=
a4x4
+
a3x3
+
a2x2
+
a1x + a0
-
Quartic
function |
|
y
=
a5x5
+ a4x4
+
a3x3
+
a2x2
+
a1x + a0
-
Quintic
function |
|
- - -
- - - - - - - - -
- - - - - - - - -
- - - - - - - - |
| ·
Rational functions - a ratio of two polynomials |
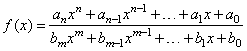 |
|
-
Reciprocal function |
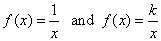 |
| |
-
Translation of the reciprocal function,
called linear rational function. |
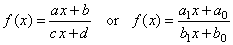 |
|
|
| The
graphs of the
polynomial functions |
| The
graph of a function ƒ
is drawing on the Cartesian plane, plotted with respect to
coordinate axes, that shows functional relationship between
variables. The points (x,
f (x)) lying on the curve
satisfy this relation. |
|
| The
source
or original polynomial function |
| Any
polynomial f(x)
of degree n >
1 in the general form, consisting
of n
+ 1 terms, shown graphically, represents translation of its
source (original) function in the direction of the coordinate
axes. |
|
The source polynomial function |
|
fs(x)
= anxn
+ an-2xn-2
+
.
. . +
a2x2
+ a1x
|
|
|
| has
n
- 1 terms
lacking second and the constant term, since its coefficients, an-1
=
0
and a0
=
0
while
the leading coefficient an,
remains unchanged. |
| Therefore,
the source polynomial function passes through the
origin. |
| A
coefficient ai
of
the source function is expressed by the coefficients of the general
form. |
|
| Translating
(parallel shifting) of the polynomial function |
| Thus,
to obtain the graph of a given polynomial function f(x)
we translate (parallel shift)
the
graph of its source function in the direction of the x-axis
by x0
and in the direction of the y-axis
by y0. |
| Inversely,
to put a given graph of the polynomial function beck to the
origin, we translate it in the opposite direction, by taking the
values of the
coordinates of translations with opposite sign. |
|
| Coordinates of translations
and their role in the polynomial expression |
| The
coordinates of translations we calculate using the formulas, |
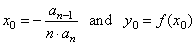
|
| Hence,
by plugging the coordinates of translations into
the source polynomial function fs(x),
i.e., |
|
y
- y0
= an(x
- x0)n
+ an-2(x
- x0)n-2
+
.
. .
+
a2(x
- x0)2
+ a1(x
- x0) |
|
|
| and
by expanding above expression we get the polynomial function in
the general form |
| f(x) =
y = anxn
+ an-1xn-1
+ an-2xn-2
+
.
. . +
a2x2
+
a1x + a0. |
| Inversely, by plugging the coordinates of translations into
the given polynomial f(x)
expressed in the general form,
i.e., |
|
y
+ y0
= an(x
+ x0)n
+ an-1(x
+ x0)n-1
+
.
. .
+ a1(x
+ x0)
+ a0 |
|
|
| and
after expanding and reducing above expression we get its source polynomial function. |
| Note
that in the above expression the signs of
the coordinates of translations are already changed. |
|
| Quartic
function y
=
a4x4
+ a3x3
+
a2x2
+
a1x + a0 |
| 1)
Calculate the
coordinates of translations by plugging
n
= 4
into |
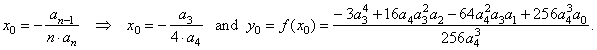
|
| 2)
To
get the source quartic function we should plug the coordinates
of translations (with changed signs) |
|
into the general form
of the quartic,
i.e., |
| y
+ y0
= a4(x
+ x0)4
+
a3(x
+ x0)3
+
a2(x
+ x0)2
+
a1(x
+ x0) +
a0, |
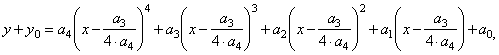 |
| after
expanding and reducing obtained is the source
quartic function |

|
| 3)
Inversely, by plugging the coordinates of translations into the source
quartic |
|
y
-
y0
=
a4(x
-
x0)4
+
a2(x
-
x0)2
+
a1(x
-
x0), |
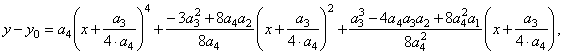
|
|
after
expanding and reducing we obtain |
|
y
= a4x4
+ a3x3
+
a2x2
+
a1x + a0
the
quartic function
in the general form. |
| Thus,
y
= a4x4
+ a3x3
+
a2x2
+
a1x + a0
or y
-
y0
=
a4(x
-
x0)4
+
a2(x
-
x0)2
+
a1(x
-
x0), |
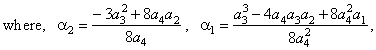 |
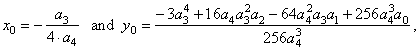 |
| by
setting x0
=
0 and
y0
= 0 we get
the source quartic y
=
a4x4
+
a2x2
+
a1x. |
|
|
|
| By
setting the coefficients a2
and a1
of the source quartic to zero, interchangeably, obtained is the
basic classification shown in the diagram. |
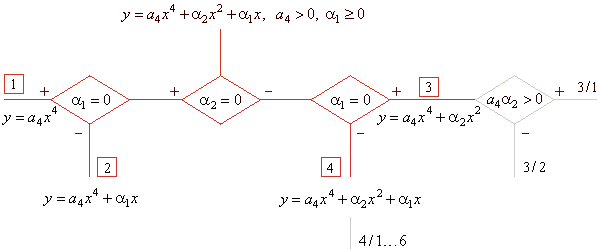 |
| There are
ten types (shapes of the graphs) of quartic functions. |
| type
1 |
y
= a4x4
+ a3x3
+
a2x2
+
a1x + a0
or y
-
y0
=
a4(x
-
x0)4,
a2
= 0 and a1
=
0. |
|
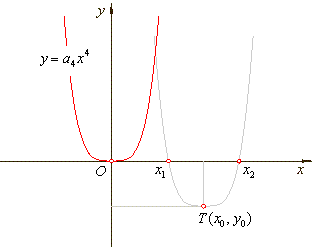 |
| The
zeroes or roots: |
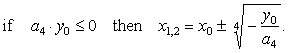 |
|
|
|
|
| type
2 |
y
= a4x4
+ a3x3
+
a2x2
+
a1x + a0
or y
-
y0
=
a4(x
-
x0)4
+ a1(x
-
x0),
a2
= 0. |
|
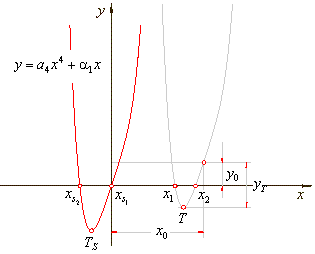 |
| The
zeroes of the source function: |
 |
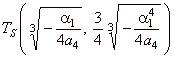 |
|
| The
zeroes of the translated function we get
by adding x0
to the solution of the
equation a4x4
+ a1x +
y0
= 0. |
 |
|
|
|
|
| type
3 |
y
= a4x4
+ a3x3
+
a2x2
+
a1x + a0
or y
-
y0
=
a4(x
-
x0)4
+ a2(x
-
x0)2,
a1
= 0. |
|
|
|
|
|
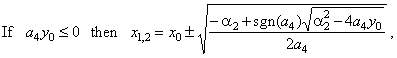 |
T
(x0,
y0). |
|
|
|
|
|
|
|
|
|
|
| Remaining
six types of quartic polynomial satisfy the criteria shown in the
diagram below. |
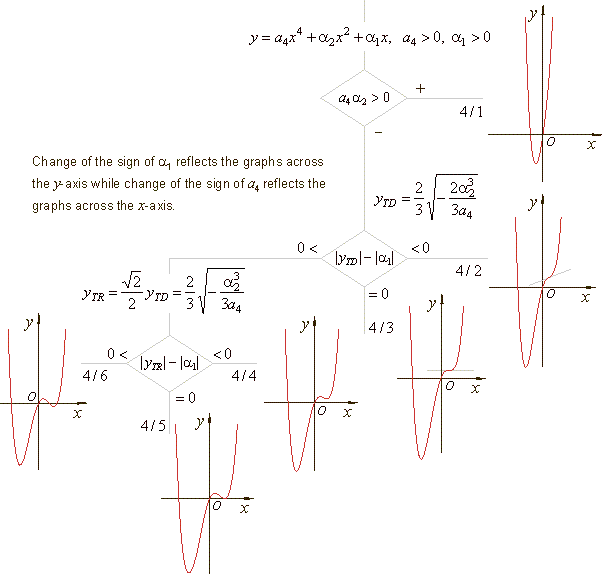 |
|
| The
roots of the source quartic
y
=
a4x4
+
a2x2
+
a1x
Types,
4/1, 4/2, 4/3 and 4/4 |
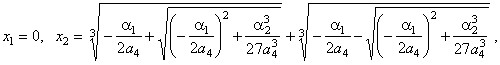 |
| the
roots of the Types,
4/5 and 4/6 |
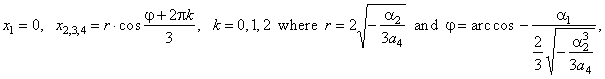 |
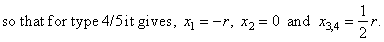 |
| The
abscissa of the turning point of the Types,
4/1, 4/2 and 4/3 |
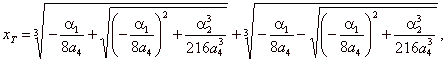 |
| the
abscissas of the turning points of the Types, 4/4,
4/5 and 4/6 |
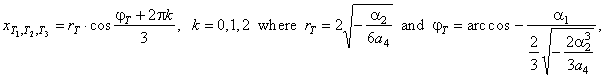 |
 |
| The
abscissas of the points of inflection of the
source quartic of Types 4/2
to 4/6, |
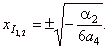 |
|
| The
roots of the translated quartic Type 4
we get by adding x0
to the solutions of the equation |
| a4x4
+
a2x2
+
a1x
+
y0
= 0. |
|
|
|
|
|
|
|