| |
|
|
Rectangular
(Two-dimensional, Cartesian) Coordinate System |
Coordinate axes, x-axis
and y-axis,
origin, quadrants
|
|
Points in the
Coordinate plane |
|
Midpoint of a line segment
|
|
The distance formula
|
|
Dividing
a line segment in a given ratio |
| |
|
|
|
|
|
| Coordinate axes, x-axis
and y-axis,
origin, quadrants
|
| The Cartesian coordinate system is defined by two
axes at right angles to each other, forming a plane. |
| The horizontal axis is
labeled x, and the vertical axis is labeled
y. |
| The point of intersection,
where the axes meet, is called the origin labeled
O. |
| Given each axis,
choose a unit length, and mark off each unit along the axis, forming
a grid. |
| The position of each point in a plane is identified with an
ordered pair of real numbers, in the form (x,
y), called the
coordinates of the point. |
| The
x-coordinate, called the
abscissa, equal to the distance of the point from the y-axis measured parallel
to the x-axis.
|
| The
y-coordinate, called the
ordinate, is the distance of the point from the x-axis measured parallel to the
y-axis.
|
| The
origin O
has coordinates (0,
0). |
| The intersection of the two axes creates four quadrants
indicated by numerals I, II, III, and IV. |
| The quadrants are labeled
counterclockwise starting from that in which both coordinates are
positive. |
 |
|
| Midpoint of a line segment
|
| The
point on a line segment that is equidistant from its endpoints
is called the midpoint. |
| The coordinates of the midpoint
M(xM,
yM)
of the line segment P1P2
where, P1(x1,
y1)
and P2(x2,
y2)
are endpoints, |
|
|
|
|
|
| Example:
Find the midpoint of the
line segment AB
where the endpoints, A(-5,
3)
and B(-1,
-1). |
|
|
|
| The distance formula
|
| The
distance between two given points in a coordinate
(Cartesian) plane. |
|
|
|
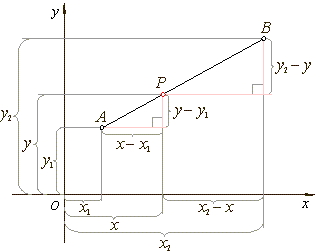
| Dividing a line segment in a given ratio
|
| A
given line segment AB
in a Cartesian plane can be divided by a point P
in a fixed ratio, internally or externally. |
| If
P
lies between endpoints then it divides AB
internally. If P
lies beyond the endpoints A
and B
it divides the segment AB
externally. |
| The
ratio of the directed segments l
=
AP
:
BP |
| is
negative in the case of the internal division since the segments
AP
and BP
have opposite sense, while in the external division, the ratio l
is positive. |
 |
|
| As
l
=
AP
:
BP |
| and
shown triangles are similar, then |
 |
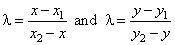| which,
with l
negative, gives |
|
|
| the coordinates of
the point P. |
| |
|
|
|
| Example:
The line segment, with
endpoints A(-3,
5)
and B(6, -1),
is divided by a point P
internally in the ratio l
= AB
: BP
= 1 : 2. Find the coordinates
of the dividing point P. |
| Solution: |
|
 |
|
|
|
|
|
|
|
 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
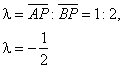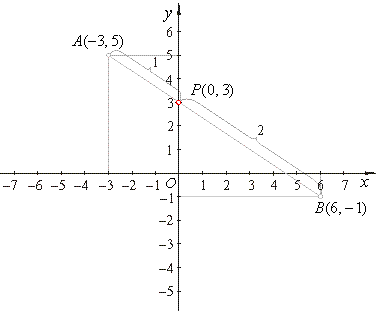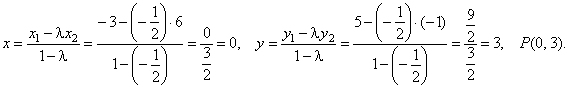
Functions
contents B
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |
