|
|
 Irrational numbers
Irrational numbers |
|
Rationalizing
a denominator |
 The
real number line, and relations
The
real number line, and relations |
|
Inequalities of real
numbers |
|
Relations,
less than and greater than |
 Interval
definition and notation
Interval
definition and notation |
|
Closed and open intervals
(unbounded
intervals) |
 Distance
and absolute value
Distance
and absolute value |
|
Properties of absolute
value |
|
|
|
|
|
|
|
|
Irrational numbers
|
|
Irrational numbers are numbers that can be written as
decimals but not as fractions.
Irrational numbers have decimal expansion that neither terminate
nor become periodic.
|
|
Any number on a number line that isn't
a rational number is irrational. For example, Ö2,
Ö3,
and Ö5
are irrational numbers because they can't be written as a ratio of two
integers. Only the square roots of square numbers are rational.
|
|
For any integers m
and n,
nÖm
is irrational unless m
is the
nth
power of an integer. Or, any
root that is not a perfect root is an irrational number.
|
|
|
| Rationalizing
a denominator |
| Rationalizing a denominator is a method for changing an irrational denominator into a rational one. |
|
|
| To
rationalize a denominator or numerator of the form |
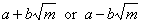 |
multiply both numerator |
|
| and denominator by a
conjugate, where |
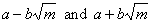 |
are conjugates of each other. |
|
|
|
|
The real number line and
relations |
|
|
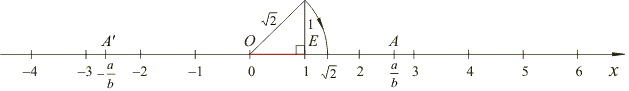 |
|
| The real number line is an infinite line on which points are taken to represent the real numbers by their distance from a fixed point labeled
O and called the
origin. |
| We
use the variable x
to denote a one-dimensional coordinate system,
in
this case the number line is called the x-axis. |
| The line segment
OE denotes
unit length, that is, |OE|
= 1. The absolute value (or modulus) of a real number
x,
denoted |x| is its numerical value without regard to its sign. |
| For example,
|+ 5| = 5 and | − 5| = 5, and 0 is the only absolute value of 0.
The absolute value of a real number a
is its distance from the origin. |
| The
unit interval is the interval [0, 1] that is the set of all real
numbers x such that
0 < x < 1, we say x
is greater than or equal to zero
and
x
is less than or equal to one, meaning x
is between 0 and 1 including the endpoints. |
| A rational number
-
a/b,
in the above picture,
corresponds to the point which is symmetrical regarding the origin to the point
A, which corresponds to the rational number
a/b . |
| Therefore,
|OA| = |OA'|
= a/b
· |OE|
= a/b. |
| For
each real number x,
there is a unique real number, denoted −x,
such that x
+ ( −x
) = 0. In
other words, by adding a number to its negative or opposite, the
result is 0. |
| Every point of the number line corresponds to one real number. |
|
| Inequalities of real
numbers |
| Relations,
less than and greater than |
Let
a
and b
are distinct real numbers. We
say that a
is less than b
if a
− b
is a negative number, and write
a
< b, i.e.,
a
< b
means a
− b
is negative. |
| On
the x-axis,
a
< b
is represented as the number a
lies to the left of b. |
| We
say that a
is greater than b
if a
− b
is a positive number, and write
a
> b, i.e.,
a
> b means a
− b
is positive. On
the x-axis,
a
> b
is represented as the number a
lies to the right of b. |
| Similarly,
a
<
b
denotes that a
is less than or equal to b,
and a
>
b,
a
is greater than or equal to b. |
|
|
Interval
definition and notation |
An
interval is the set containing all real numbers (or points)
between two given real numbers, a
and
b,
where
a
< b. |
A
closed interval [a,
b]
includes the endpoints, a
and
b,
and it corresponds to a set notation
{x
| a
<
x
<
b},
while an open interval (a,
b) does
not include the endpoints. |
| On
the real line, the half-closed (or half-opened)
interval
from a
to b
is written [a,
b)
or (a,
b],
where square brackets indicate inclusion of the endpoint, while
round parentheses denote its exclusion. |
| Thus,
[a,
b]
= {x
| a
<
x
<
b}
- a closed interval |
|
[a,
b)
= {x
| a
<
x
< b}
- an interval closed on left, open on right |
|
(a,
b]
= {x
| a
< x
<
b}
- an interval open on left closed on right |
|
(a,
b)
= {x
| a
<
x
< b}
- an open interval |
| Unbounded
intervals or intervals of infinite length are also written
in this notation, thus [a,
oo ) is unbounded interval x
>
a,
which is regarded as closed, while (a,
oo
) is the open interval x
> a. |
|
Hence,
(
−oo
, a]
= {x
| x
<
a}
and [a,
oo
)
= {x
| x
>
a},
|
|
(
−oo
, a)
= {x
| x
< a}
and (a,
oo
)
= {x
| x >
a}.
|
|
The real line R
= (
−oo
, oo
).
|
|
|
| Distance
and absolute value |
| The
distance between a number x
and 0 equals x
if x
>
0, and equals −x
if x
< 0,
therefore the absolute value of a number x, denoted
| x |, is x
if x
>
0, and −x
if x
< 0. |
| From
the definition of the absolute value it follows that the
distance between two numbers a
and b, |
|
|
| |
| Properties of absolute value |
Examples |
| 1 |
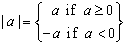 |
1 |
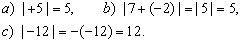 |
| 2 |
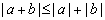 |
2 |
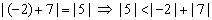 |
| 3 |
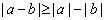 |
3 |
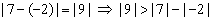 |
| 4 |
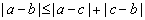 |
4 |
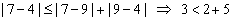 |
| 5 |
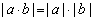 |
5 |
 |
| 6 |
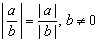 |
6 |
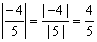 |
| 7 |
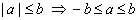 |
7 |
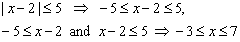 |
| 8 |
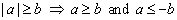 |
8 |
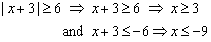 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| Functions
contents A |
|
|
 |
|
| Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved. |