|
|
|
|
Analytic geometry
- Conic sections
|
|
|
|
Construction of the tangent at the point on the parabola and from a point exterior to the parabola
|
|
Construction of the tangent at the point on the parabola
|
|
As the vertex of the parabola is the midpoint of the line
segment whose endpoints are, the projection P1'
|
|
of the given point to the axis of the parabola and the point
B opposite it, draw the tangent through points B and P1.
|
|
Construction of the tangents from a point exterior to the parabola
|
|
Draw the circle centered at the point A
outside the parabola through the focus.
|
| The circle intersects the directrix at
points, |
| D1
and D2.
|
|
Tangents from the point
A
are then perpendicular
bisectors of the line segments, D1F
and D2F.
|
| Thus, the tangency points,
P1
and P2
are equidistant from the focus and the directrix. |
|
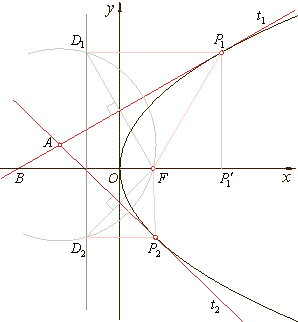 |
|
|
Parabola and
line examples
|
|
Example:
Find the point on the parabola
y2 = 9x
closest to the line 9x + 4y + 24 =
0.
|
|
Solution: The tangency point of the tangent parallel to
the given line is the closest point.
|
| 9x + 4y +
24 = 0, y =
-(9/4)x
-
6, mt =
-
9/4
|
|
The slope of the tangent must satisfy tangency condition
of the parabola,
|
|
p = 2mc
<= mt =
-
9/4, p
=
9/2
|
|
9/2 = 2 · (-9/4)
· c
=>
c
= -
1
|
|
therefore, the
tangent t
:: y =
-(9/4)x
-
1.
|
|
The solution
to the system of equations of the tangent and the parabola
gives the tangency point, that
is
|
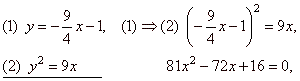
|
|
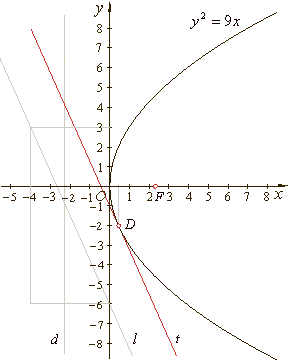 |

|
|
|
Example:
Given is the polar
4x + y + 12 =
0 of the parabola
y2 = -4x, find coordinates of the pole and
write equations of the corresponding tangents.
|
|
Solution: Intersections of the polar and the parabola are the
tangency points of tangents drawn from the pole P.
Thus, by solving the system of equations of the polar and the parabola
we get tangency points.
|
|
|
|
The intersection of tangents is the pole P.
Therefore, we solve the system formed by their equations, |
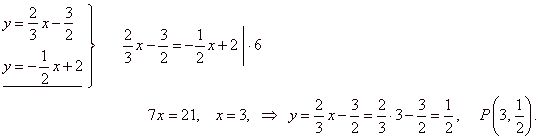 |
|
|
|
Conics, a
family of similarly shaped curves
- properties of conics
|
|
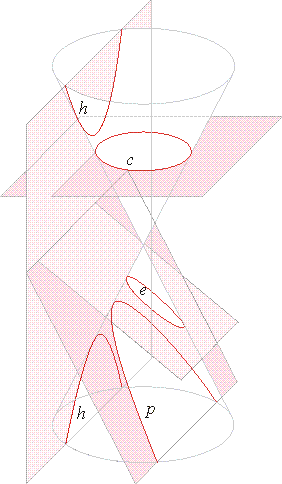
By intersecting
either of the two right circular conical surfaces (nappes) with the plane
perpendicular to the
axis of
the cone the resulting intersection is a circle
c, as
is shown in the right figure. |
|
When the cutting plane is inclined to the axis of the cone at a greater angle than that made by
the generating segment or generator (the
slanting edge of the cone), i.e., when the plane cuts all
generators of a single cone, the resulting curve is the
ellipse
e.
|
| Thus, the circle is a special case
of the ellipse in which the plane is perpendicular to the axis of the cone.
|
|
If the cutting plane is parallel to any generator of one of the cones, then the intersection curve is
the
parabola
p.
|
|
When the cutting plane is inclined to the axis at a smaller angle than the generator of the cone,
i.e., if the intersecting plane cuts both cones the
hyperbola
h
is generated.
|
|
 |
|
|
::
Dandelin
spheres proof of conic sections focal properties
|
|
Proof that conic section curve is
the ellipse
|
|
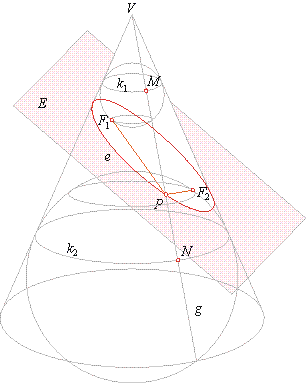
In the case when the plane
E
intersects all generators of the cone, as in down figure, it is possible
to inscribe two spheres which will touch the conical surface and the plane. |
|
Upper sphere touches the cone surface in a circle
k1
and the plane at a point F1.
Lower sphere touches the cone surface in a circle
k2
and the plane at a
point
F2. Arbitrary chosen generating line
g
intersects
|
| the
circle k1
at a point
M, the circle
k2
at a point
N
and the intersection curve e
at a point
P.
|
| The
points,
M
and F1
are the tangency points of the upper sphere and points, N
and F2
are the tangency points of the lower sphere of the tangents
drawn from the point P
exterior to the spheres.
|
| Since the segments of tangents from a point
exterior to sphere to the points of contact, are equal
|
| PM =
PF1
and PN =
PF2.
|
|
And since planes of
circles k1and
k2,
are parallel, then are all corresponding generating segments equal |
| MN =
PM +
PN
is constant.
|
|
Thus, the intersection curve is the locus of points
in the plane for which sum of distances from the
two fixed
points F1and
F2, is constant, i.e., the curve is the ellipse.
|
|
 |
|
| The proof due to the French/Belgian mathematician Germinal Dandelin (1794 –
1847). |
|
|
Conics, a family of similarly shaped curves
|
|
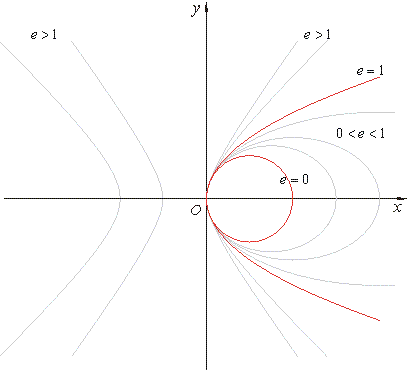
A conic is the set of points
P
in a plane whose distances from a fixed point F
(the focus) and a fixed line d
(the directrix), are in a constant ratio. This ratio named the
eccentricity e
determines the shape of the curve. |
| We can see that conics represent a family of similarly shaped curves if we write their equations in vertex
form. Recall the method we used to transform equations of the ellipse and the hyperbola from standard to vertex
form. We placed the vertex of the curve at the origin translating its graph. |
|
|
The
equation of conics in the vertex form
|
|
Thus, obtained are their vertex
equations |
| y2 =
2px -
(p/a)x2 |
| -
the
ellipse
and the
circle |
| (for
the circle p =
a = r) |
|
|
|
|
|
| y2 =
2px + (p/a)x2 |
| -
the
hyperbola |
|
|
|
|
In
the above vertex equations we can make following substitutions for, |
|
 |
|
| the ellipse |
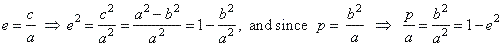 |
|
| the
circle |
p =
a = b = r
=> e
= 0 |
|
|
|
| the
hyperbola |
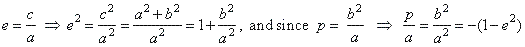 |
|
|
Thus, the
equation of conics in vertex form is
y2 =
2px -
(1 -
e2)x2.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Contents
D
|
|
|
|
|

|
|
|
|
|
|
Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved.
|
|
|