29
 
Analytic geometry - Conic sections
 ::  Intersection of ellipse and line - tangency condition

 Common points of a line and an ellipse we find by solving their equations as a system of two equations in two unknowns, x and y,
   (1)  y = mx + c                     by plugging (1) into (2)    =>      b2x2 + a2(mx + c)2 =  a2b2
   (2)  b2x2 + a2y2 = a2b2         and after rearranging   (a2m2 + b2)·x2 + 2a2mc·x + a2c2 - a2b2 = 0
obtained is the quadratic equation in x. Thus, the coordinate of intersections are,
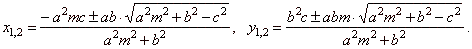Using the above solutions follows that a line and an ellipse can have one of three possible mutual positions depending of the value of the discriminant  D = a2m2 + b2 - c2. Thus, if
        D > 0,  a line and an ellipse intersect,  and if    D < 0,  a line and an ellipse do not intersect,

while if   D = 0,  or  a2m2 + b2 = c2  a line is the tangent to the ellipse thus, it is the tangency condition.
The line touches the ellipse at the tangency point whose coordinates are:
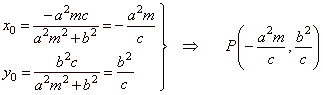Equation of the tangent at a point on the ellipse

In the equation of the line  y - y1 = m(x - x1) through a given point P1, the slope m can be determined using known coordinates (x1, y1) of the point of tangency, so

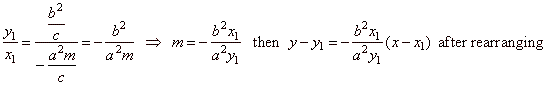b2x1x + a2y1y = b2x12 + a2y12, since  b2x12 + a2y12 = a2b2  is the condition that P1 lies on the ellipse

then  b2x1x + a2y1y = a2b2  is the equation of the tangent at the point P1(x1, y1) on the ellipse.

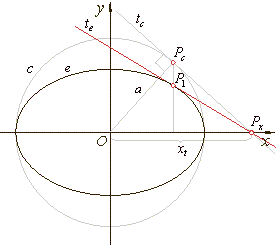
Construction of the tangent at a point on the ellipse

Draw a circle of a radius a concentric to the ellipse. Extend the ordinate of the given point to find

intersection with the circle. The tangent of the circle at Pc  intersects  the x-axis at Px. The tangent to the ellipse at the point  P1 on the ellipse intersects the x-axis at the same point.

To prove this, find the x-intercept of each tangent       
analytically.

Therefore, in both equations of tangents set  y = 0 and 
solve for x,
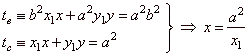it is the x-intercept of the tangent tc and the tangent te.
Angle between the focal radii at a point of the ellipse

Let prove that the tangent at a point P1 of the ellipse is perpendicular to the bisector of the angle between the focal radii r1 and  r2.  Coordinates of points, F1(-c, 0), F2(c, 0) and P1(x1, y1) plugged into

the equation of the line through two given points define the lines of the focal radii

r1 = F1P1  and   r2 = F2P1,
and the equation of the tangent at the point P1,

By plugging the slopes of these tree lines into the formula for calculating the angle between lines we find the exterior angles j1 and j2 subtended by these lines at P1.

Thus, using the condition b2x12 + a2y12 = a2b2, that the point lies on the ellipse, obtained is

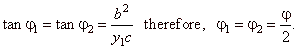Therefore, the normal at the point P1 of the ellipse bisects the interior angle between its focal radii.

Example:  Find a point on the ellipse x2 + 5y2 = 36 which is the closest, and which is the farthest from the line 6x + 5y - 25 = 0.

Solution:  The tangency points of tangents to the ellipse which are parallel with the given line are, the 
closest and the farthest points from the line. Rewrite the equation of the ellipse to determine its axes,

Tangents and given line have the same slope, so
Using the tangency condition, determine the intercepts c
therefore, the equations of tangents,

Solutions of the system of equations of tangents to the ellipse determine the points of contact, i.e., the 
closest and the farthest point of the ellipse from the given line, thus
 Tangents to an ellipse from a point outside the ellipse - use of the tangency condition

Coordinates of the point A(x, y), from which we draw tangents to an ellipse, must satisfy equations of the tangents,  y = mx + c and their slopes and intercepts, m and c, must satisfy the condition of tangency therefore, using the system of equations,
    (1)  y = mx + c     <=    A(x, y)

    (2)  a2m2 + b2 = c2  determined are equations of the tangents from a point A(x, y) outside the ellipse.
 Construction of tangents from a point outside the ellipse

With A as center, draw an arc through F2, and from F1 as center, draw an arc of the radius 2a. Tangents are then the perpendicular bisectors of the line segments, F2S1 and F2S2. 

We can also draw tangents as lines through A and the intersection points of the segments F1S1 and F1S2 and the ellipse.

Thus, these intersections are the tangency points of the tangents to the ellipse.
Explanation of the construction lies at the fact that,
F1S1 = F1D1 + D1S1 = F1D1 + D1F2 = 2a
according to the definition of the ellipse, as well as the point A is equidistant from points F2 and S1, since the point S1 lies on the arc drawn from A through F2.

Example:  Determine equation of the ellipse which the line -3x + 10y = 25 touches at the point               P(-3, 8/5).
Solution:  As the given line is the tangent to the ellipse, parameters, m and c of the line must satisfy the tangency condition, and the point P must satisfy the equations of the line and the ellipse, thus

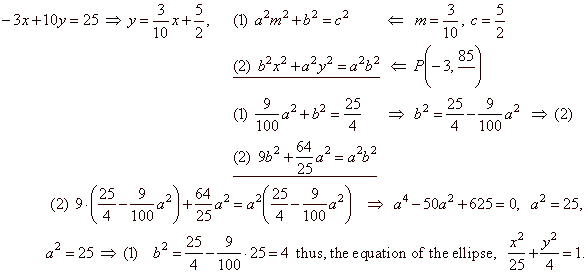Example:  Find the equations of the common tangents of the curves  4x2 + 9y2 = 36 and  x2 + y2 = 5.

Solution:  The common tangents of the ellipse and the circle must satisfy the tangency conditions of these curves, thus
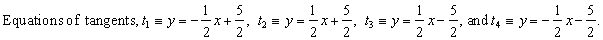 Polar and pole of the ellipse

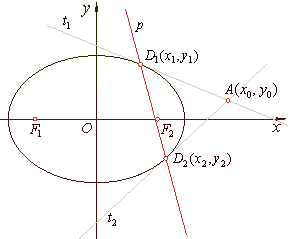
If from a point A(x0, y0), exterior to the ellipse, drawn are tangents, then the secant line passing through the contact points, D1(x1, y1) and D2(x2, y2) is the polar of the point A. The point A is called the pole of 
the polar, as shows the right figure.

Coordinates of the point A(x0, y0) must satisfy the  equations of tangents, thus
t1 ::   b2x0x1 + a2y0y1 = a2b2
t2 ::   b2x0x2 + a2y0y2 = a2b2
and after subtracting t1 - t2
b2x0(x2 - x1) + a2y0(y2 - y1) = 0
obtained is
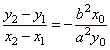the slope of the secant line through points of contact D1and D2.
Thus, the equation of the secant line 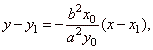and after rearranging
b2x0x + a2y0y = b2x0x1 + a2y0y1,  since   b2x0x1 + a2y0y1 = a2b2
follows     b2x0x + a2y0y = a2b2     the equation of the polar p of the point A(x0, y0).

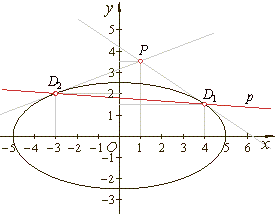
Example:  The line x + 14y - 25 = 0 is the polar of the ellipse x2 + 4y2 = 25.  Find coordinates of the pole.

Solution:  Intersections of the polar and the ellipse are points of contact of tangents drawn from the pole P to ellipse, thus solutions of the system of equations,
(1)  x + 14y - 25 = 0   =>  x = 25 - 14y  =>  (2)
  (2)  x2 + 4y2 = 25
 (25 - 14y)2 + 4y2 = 25
2y2 - 7y + 6 = 0,      y1 = 3/2 and  y2 = 2
y1 and y2  =>  x = 25 - 14y,   x1 = 4 and  x2 = -3.
Thus, the points of contact  D1(4, 3/2) and D2(-3, 2).
The equations of the tangents at D1 and D2,
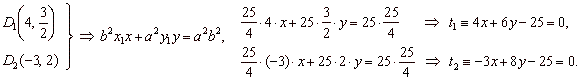The solutions of the system of equations t1 and t2 are the coordinates of the pole P(1, 7/2).
 
 
 
 
 
 
 
Contents C
 
 
 
 
Copyright © 2004 - 2020, Nabla Ltd.  All rights reserved.