|
|
|
|
Analytic geometry
- Conic sections
|
|
|
|
Ellipse
|
|
::
Ellipse,
definition and construction, eccentricity and linear
eccentricity
|
|
By intersecting
either of the two right circular conical surfaces (nappes) with the plane inclined to the axis of the cone at a greater angle than that made by
the generating segment or generator (the slanting edge of the cone), i.e., when the plane cuts all
generators of a single cone, the resulting curve is the ellipse
e.
|
|
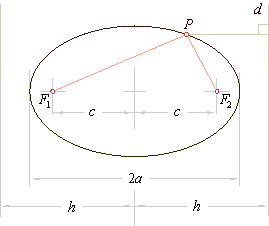
An ellipse is the set of points (locus) in a plane whose distances from two fixed points have a constant sum.
The fixed points F1
and F2 are called foci. Thus, the sum of distances
of any point P, of the ellipse,
|
|
from the
foci is |
F1P |
+
|
F2P | =
2a, and the
distance between foci |
F1F2 | =
2c, |
|
where 2a is the
major
axis,
2c is called
focal distance and
c
is also called linear
eccentricity. |
|
Quantities
a
and c,
a
> c,
uniquely determine an ellipse. The ratio e = c/a,
e < 1
is called eccentricity
of the ellipse. |
| The ellipse can also be defined as the locus of points the ratio of whose distances from the
focus to a vertical line, known as the
directrix ( d
), is a constant
e,
where |
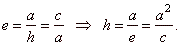 |
|
 |
|
|
|
Constructions of an ellipse
|
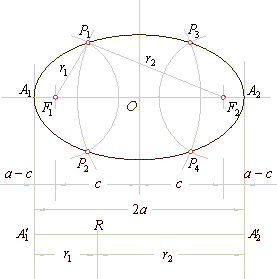
| On a given line segment
A1'
A2'
= 2a, of the major
axis, choose an arbitrary point R.
|
|
Draw an arc of the radius A1'R
= r1
with center F1
and then draw an arc with center F2
and radius A2'R
= r2, intersecting the arc at points P1 and
P2.
|
|
Repeat the same procedure by drawing the arc of the radius
r1
centered at F2
and the arc of the radius
r2
with the center F1 to obtain intersections
P3 and
P4.
|
|
Using this method we can draw as many points of the ellipse as needed, noticing that while choosing point R, always must be
r1
> a -
c and r2
>
a -
c.
|
|
This construction shows that the ellipse has two axes of symmetry of different length, the
major and
minor axes. Their intersection is the center of the ellipse.
|
|
 |
|
|
|
Equation of the ellipse, standard
equation of the ellipse
|
| If in the direction of axes we introduce a coordinate system so that the center of the ellipse coincides with |
| the
origin, then coordinates of foci are |
| F1(-c, 0) and
F2(
c, 0). |
| For every
point P(x,
y) of the ellipse, according to
the |
| definition
r1 + r2 =
2a, it follows
that |
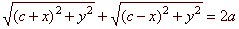 |
| after
squaring |
|
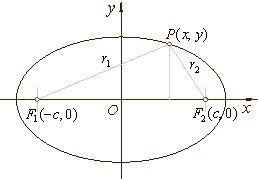 |
|
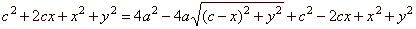 |
| and
reducing |
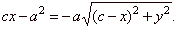 |
|
| Repeated squaring and grouping gives |
| (a2
-
c2) · x2
+ a2y2 = a2
· (a2 -
c2), |
|
and
since a2
-
c2 = b2 |
|
follows b2x2
+ a2y2 = a2b2
equation
of the ellipse, |
|
 |
|
| and after division by
a2b2, |
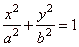 |
standard equation of the ellipse. |
|
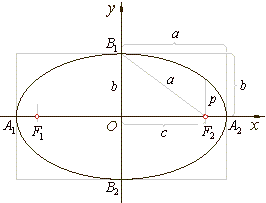
The line segments
A1A2
= 2a and
B1B2
= 2b are the
major and
minor axes while
a and b
are the
semi-major and
semi-minor axes respectively. So the arc of the radius
a
centered at B1and
B2 intersects the major axis at
the foci F1and
F2. |
|
The focal parameter, called latus rectum and denoted
2p, is the chord perpendicular to the major axis
passing through any of the foci, as shows the above figure. |
| The length of the latus rectum |
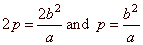 |
the
length of the semi-latus rectum of the ellipse. |
|
|
|
Example:
Given is equation of the ellipse
9x2
+ 25y2 = 225, find the lengths of semi-major and
semi-minor axes, coordinates of the foci, the eccentricity and the length of the
semi-latus rectum.
|
|
Solution: From the standard equation |
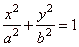 |
we can find the semi-axes
lengths dividing the given |
|
| equation by
225, |
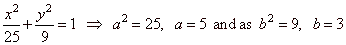 |
|
| coordinates of the
foci F1(-c, 0) and
F2(
c, 0),
since
|
 |
 |
|
|
Example:
Write equation of the ellipse passing through points
A(-4,
2) and B(8,
1).
|
|
Solution: Given points must satisfy equation of the ellipse, so |
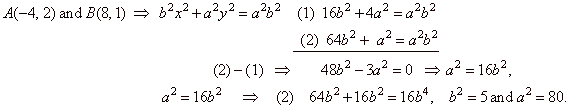 |
| Therefore,
the equation of the ellipse |
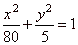 |
or
x2
+ 16y2 = 80. |
|
|
|
The parametric equations of the
ellipse
|
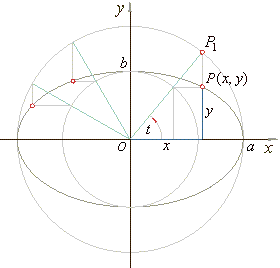
| Equation of the ellipse in the explicit form |
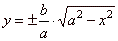 |
help us to explain another construction |
|
|
of
the ellipse. So, in the coordinate system draw two concentric circles of radii equal to lengths of the semi axes a and b, with the center at the origin as shows the figure.
|
|
An arbitrary chosen line through the origin intersects the
circle of the radius a
at the point R
and the circle of radius b
at M.
|
|
Then, the parallel line with the major axis through M
intersects the parallel line with the minor axis through
R, at
a point P(x,
y) of the ellipse.
Proof,
|
| in
the figure, OS
= x,
PS
= y
and |
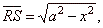 |
|
|
as the triangles
OMN
and ORS
are similar, then
|
| OM
: OR
= MN
: RS
or b
: a
= PS
: RS,
|
| so
that |
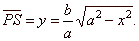 |
|
|
 |
|
| It proves that the point
P(x,
y) obtained by the construction
lies on the ellipse. This way, using the figure, we also derive |
| the
parametric equations of
the ellipse |
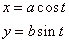 |
where the parameter
t
is an angle 0
<
t
<
2p. |
|
|
By dividing the first parametric equation by
a
and the second by b, then square and add them, obtained is
standard equation of the ellipse.
|
|
|
Equation of a translated ellipse
|
|
The equation of an ellipse that is translated from its standard position can be obtained by replacing
x
by
|
| x0
and
y by
y0 in its standard equation, |
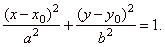 |
|
|
The above equation can be rewritten into
Ax2
+ By2 + Cx + Dy + E = 0.
|
|
Every equation of that form represents
an ellipse if A
not equal
B
and A ·
B > 0 that is, if the square terms have unequal coefficients, but the same
signs.
|
|
|
Example:
Given is equation of the ellipse
4x2
+ 9y2
+ 24x -18y
+ 9 =
0,
find its center S(x0,
y0),
the semi-axes and intersections of the ellipse with the coordinate axes.
|
|
Solution: Coordinates of the center and the semi-axes are shown
in the equation of the translated ellipse, |
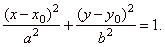 |
| Rewrite the given equation to that
form, |
| 4(x2
+ 6x)
+ 9(y2 -
2y)
+ 9 = 0 |
|
4[(x + 3)2 -
9] +
9[(y -1)2
-1]
+ 9 = 0
|
|
4(x + 3)2
+ 9(y -1)2
= 36
or
|
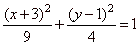 |
|
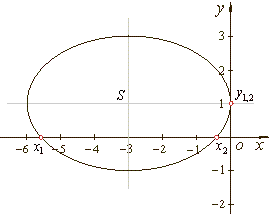 |
|
| therefore,
S(-3,
1),
a = 3 and
b = 2.
|
|
Intersections of the ellipse and the
x-axis
we obtain by setting y =
0 into the equation of the ellipse, thus
|
| 4x2
+ 24x + 9 =
0,
x1,2 = -3
± 3Ö3/2, |
|
and intersections of the ellipse
with the y-axis by
setting x
= 0, =>
9y2
-18y
+ 9 =
0,
y1,2 = 1. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Contents C
|
|
|
|
|

|
|
|
|
|
|
Copyright
© 2004 - 2020, Nabla Ltd. All rights reserved.
|
|
|