21
 
Coordinate geometry (or Analytic geometry) in three-dimensional space
 Points, lines and planes in three-dimensional coordinate system represented by vectors
 :: Equations of a line in space - The vector's equation of a line

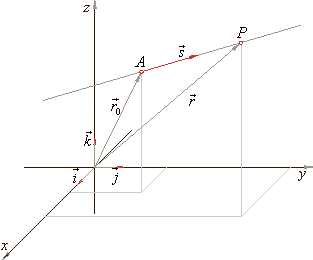
  Through a given point A(x0, y0, z0), which is determined by position vector r0 = x0i + y0 j + z0k, passes a line directed by its direction vector s = ai + bj + ck.

Thus, the position of any point P(x, y, z) of a line is then uniquely determined by a vector

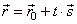
which is called the vector's equation of a line.

 That is, a radius vector  r = xi + y j + zk

of every point of the line, represents the sum of the radius vector r0, of the given point, and a vector  t · s  collinear to the vector s, where t is a parameter which can take any real value from       - oo  to + oo.

The parametric equations of a line
By writing the above vector equation of a line in the component form
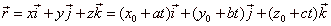
obtained are components of the vector r,    x = x0 + at,   y = y0 + bt  and  z = z0 + ct
which, at the same time represent coordinates of any point of the line expressed as the function of a variable parameter t. That is why they are called the parametric equation of a line.
Equation of a line defined by direction vector and a point - Symmetric equation of a line
Let express t from the above parametric equations
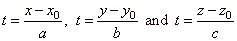then, by equating obtained is
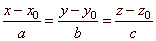equation of a line passing through a point A(x0, y0, z0) and given direction vector s = ai + bj + ck.
Scalar components (or the coordinates), a, b, and c, of the direction vector s, are
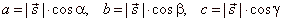or the direction cosines

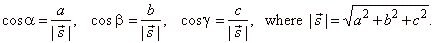That is, the cosines of the angles that a line forms by the coordinates axes x, y and z, or the scalar components of the unit vector of the direction vector s

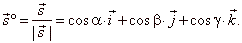Line given by two points

A line through points A and B, determined by their position vectors,
 r1 = x1i + y1 j + z1k  and  r2 = x2i + y2 j + z2k,
has the direction vector s = r2 - r1 so that its vector equation is
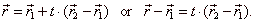If we write this equation in the component form that is
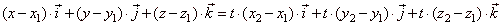by equating corresponding scalar components
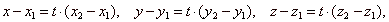and by eliminating parameter t
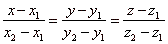obtained is equation of a line through two given points, A(x1, y1, z1) and B(x2, y2, z2).
The direction cosines are,
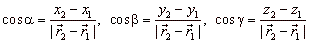where
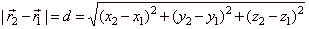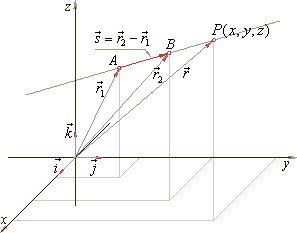is the distance between given points A and B.
If, for example, in the above equation of a line through two points in a space we set  z coordinate of both given points zero, we obtain known equation of a line through two points in a coordinate plane, i.e.,

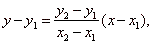and at the same time, it is the equation of the orthogonal projection of a line in 3D space onto the xy  coordinate plane.

Example:  Determine equation of a line passing through the point A(-1, -2, 3) and which is parallel to the vector s = 2i + 4j + 2k.
Solution:

By plugging coordinates of the given point                   A(x0, y0, z0) and the components a, b and c, of the direction vector s = 2i + 4j + 2k, into equation of a line,
obtained is
Example:  Find the angles that a line 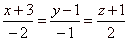forms with coordinate axes.
Solution:  The unit vector of the direction vector s = -2i - j + 2k
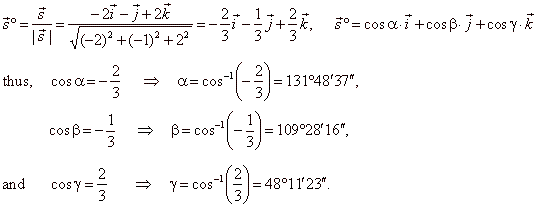Angle between lines
Angle between two lines
equals the angle subtended by direction vectors, s1 and s2 of the lines
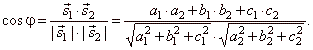For the lines that do not intersects, i.e., for the skew lines (such as two lines not lying on the same plane in space), assumed is the angle between lines that are parallel to given lines that intersect.
That is, the initial points of their direction vectors always can be brought to the same point by translation.
Condition for intersection of two lines in a 3D space
Two lines in a 3D space can be parallel, can intersect or can be skew lines.

Two parallel or two intersecting lines lie on the same plane, i.e., their direction vectors, s1 and s2 are coplanar with the vector P1P2 = r2 - r1 drawn from the point P1, of the first line, to the point P2 of the second line.
Therefore, the scalar triple product of these vectors is zero,
 
 
 
 
 
 
 
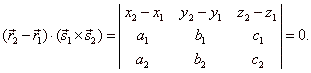Contents C
 
 
 
 
Copyright © 2004 - 2020, Nabla Ltd.  All rights reserved.