Vectors and a coordinate system, Cartesian vectors, Vectors in three-dimensional space in terms of Cartesian coordinates

ALGEBRA

:: Vectors and a coordinate system,Cartesian vectors

Vectors in a coordinate plane (a two-dimensional system of coordinates), Cartesian vectors

By introducing a coordinate system in a plane with the unit vectors, i and j (in direction of x and y coordinate axis, respectively) whose tails are in the origin O, then each point of the plane determines a vector r = OP.

A directed line segment from the origin to a point P (x, y) in plane is called a radius vector and denoted r.

The radius (or position) vector equals the sum of its vector components, xi and y j in direction of coordinate axes, that is

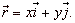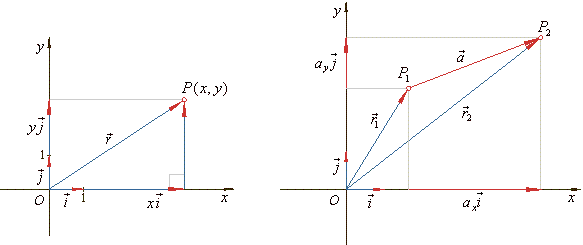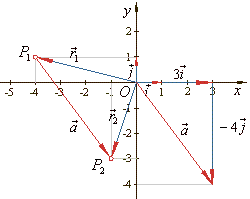
Consider a vector a in the plane directed from a point P₁(x₁, y₁) to P₂(x₂, y₂) shown in the right diagram, it also equals to the sum of corresponding vector components a_xi and a_yj, in direction of coordinate axes,

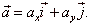The diagram shows that

are the radius vectors of points P₁ and P₂,

thus the vector a equals to the difference (joins their heads), that is

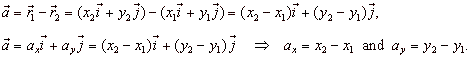It is obvious from the above diagrams that a vector and its components, i.e., its projections in direction of
coordinate axes, form a right triangle, from which, according to Pythagoras’ theorem, we determine the length of the vector,

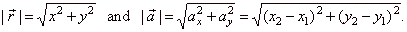Example: Determine a vector a whose tail is at the point P₁(-4, 1) and head at the point P₂(-1, -3).

Solution: Points, P₁ and P₂ determine radius vectors,

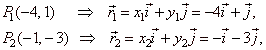therefore,

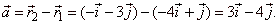Vectors in three-dimensional space in terms of Cartesian coordinates

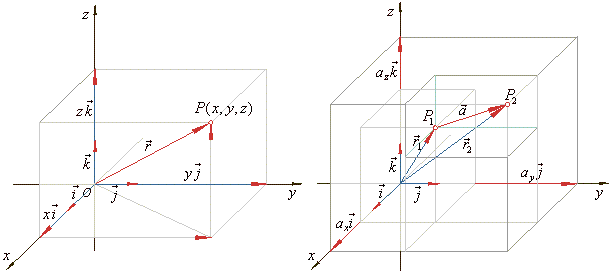
By introducing three mutually perpendicular unit vectors, i, j and k, in direction of coordinate axes of the
three-dimensional coordinate system, called standard basis vectors, every point P(x, y, z) of the space
determines the radius vector or the position vector,

and

the length of

Similarly, a vector a in the right diagram, which is directed from a point P₁(x₁, y₁, z₁) to a point P₂(x₂, y₂, z₂) in space, equals to sum of its vector components, a_xi, a_yj, and a_zk, in the direction of the coordinate axes, x, y, and z respectively, that is

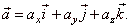The vector a is the difference of the radius vectors,

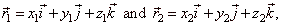thus

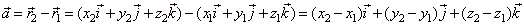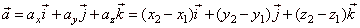so the scalar (numeric) components of the vector a,

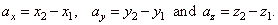Therefore, the length of the vector a,

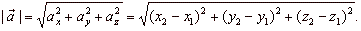Angles of vectors in relation to coordinate axes, directional cosines - scalar components of a vector

The scalar components of a vector and its magnitude form a right triangle in which the hypotenuse equals the magnitude of the vector, then

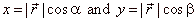since b = 90° - a then cosb = sina,

and components of a vector a,

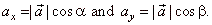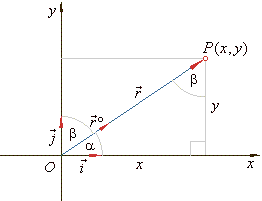If a vector a in 3D space forms with the coordinate axes, x, y and z angles, a, b and g respectively, then

components of the vector are,

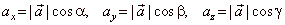while for the radius vector of the point P(x, y, z),

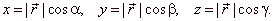Thus, for angles, a, b and g hold the expressions,

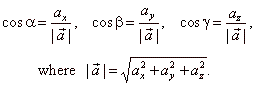called the directional cosines of the vector a.

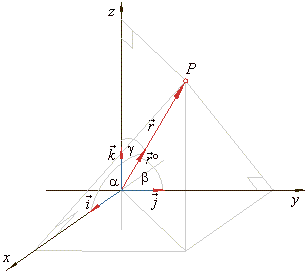The unit vector of the vector a,

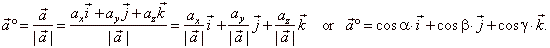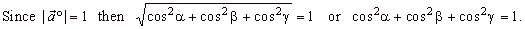This condition must satisfy angles that a vector in three-dimensional space form with the coordinate axes.

For vectors in a coordinate plane

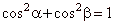and since b = 90° - a, then

cosb = cos(90° - a) = sina, follows

the basic trigonometric identity.

Similarly, the unit vector of a radius vector in space

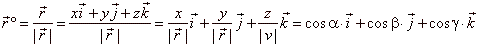determined by corresponding directional cosines.

Therefore the radius vector forms with coordinate axes angles,

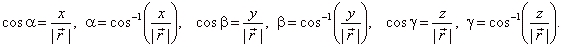As vectors are uniquely determined by its components or coordinates, they are usually denoted using matrix algebra notation, in the coordinate plane,

and in the 3-D space

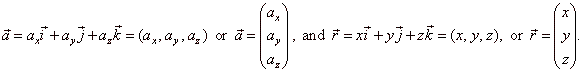Vectors in a three-dimensional coordinate system, examples

Example: Determine angles that a radius vector of the point A(3, -2, 5) forms with the coordinate axes.

Solution: Let calculate the magnitude or length of the radius vector,

Angles between the radius vector and the coordinate axes are,

Example: A vector a in a 3D-space, of the length | a | = 4, forms with axes, x and y the same angles, a = b = 60°, find the components (coordinates) of the vector a.

Solution: Using relation
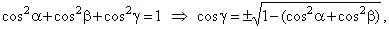applying given conditions,
The components of a,

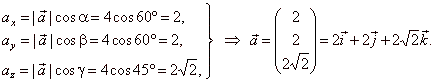Example: Prove that vectors, a = -i + 3 j + k, b = 3i - 4 j - 2k and c = 5i - 10 j - 4k are coplanar.

Solution: If all three vectors lie on the same plane then there are coefficients, l and m such that, for example c = la + mb, i.e., each of the vectors can be expressed as the linear combination of the remaining two.

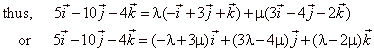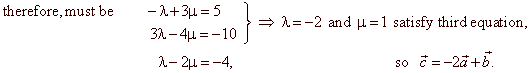Contents B