|
|
Vectors in a Plane and Space |
|
Vectors
and a coordinate system, Cartesian vectors |
|
Square of magnitude of
a vector |
|
Scalar product of unit
vectors |
|
Scalar or dot product
properties |
|
Scalar product in the
coordinate system |
|
Vectors examples |
|
|
|
|
|
|
|
| Square of magnitude of
a vector |
| The scalar product of a vector with itself,
a ·
a
= a2
is the square of magnitude of a vector, that is |
|
|
| thus, |
 |
-
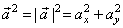in the coordinate plane, |
and |
 |
-
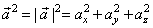in 3D space. |
|
|
| Scalar product of unit
vectors |
| The unit vectors,
i,
j and k,
along the Cartesian coordinate axes are orthogonal and their scalar products are, |
|
|
|
| Scalar or dot product
properties |
| a)
k
· (a · b)
= (k · a) · b
= a · (k · b),
k
Î
R |
| b)
a
· b = b · a |
| c)
a
· (b + c)
= a · b + a
· c |
| d)
a
· a = a2
= | a |2 |
|
 |
|
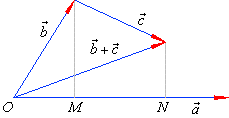| According to the definition of the dot product, from the
above diagram, |
 |
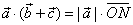then |
|
|
 |
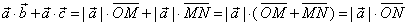what confirms the distribution law. |
|
|
|
|
The associative law does not hold for the dot product of more vectors, for example |
|
a
· (b · c) is
not equal (a
· b) · c |
| since
a
· (b · c) is the vector
a
multiplied by the scalar b
· c,
while (a
· b) · c
is the vector c
multiplied by the scalar a
· b. |
|
| Scalar product in the
coordinate system |
| The scalar product can be expressed in terms of the
components of the vectors, |
 |
| |
 |
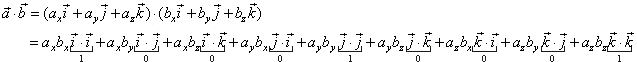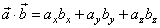- the scalar product in three-dimensional coordinate system, |
|
| |
 |
- the scalar product in the coordinate plane. |
|
|
| Vectors
examples |
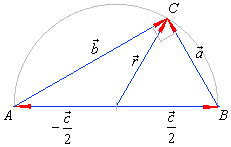
| Example:
Applying the scalar product, prove
Thales’ theorem which states that an angle inscribed in a |
| semicircle is a right angle. |
| Solution:
According to diagram in
the right figure |
 |
 |
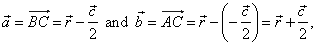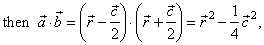| since square of a vector equal to square of its length, |
|
 |
|
 |
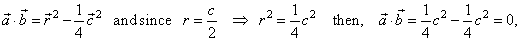| thus,
a
and b are orthogonal vectors as |
 |
. |
|
|
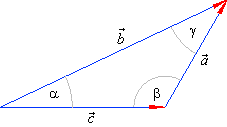
| Example:
Prove the law of cosines used in the trigonometry of oblique triangles. |
| Solution:
Assuming the directions of vectors as in
the right diagram |
 |
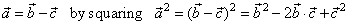Using the scalar product and substituting square of vectors
by square of their lengths, obtained is |
| a2 =
b2 + c2 -
2bc · cosa
- the law of cosines. |
|
 |
|
| In the same
way, |
 |
|
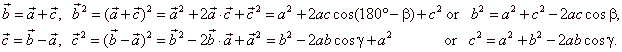| Example:
Determine a parameter l so the given vectors,
a
= -2i
+ l
j
-
4k
and b
= i -
6 j + 3k
to be perpendicular. |
| Solution:
Two vectors are perpendicular if their scalar product is zero, therefore |
 |
|
| Example:
Find the scalar product of vectors, a
= -3m
+ n
and b
= 2m -
4n if
| m |
= 3 and
| n |
= 5 , and the
angle between vectors, m
and n is
60°. |
|
|
|
| Example:
Given are vertices, A(-2,
0, 5), B(-3, -3,
2), C(1,
-2,
0) and
D(2,
1, 3), of a parallelogram, |
| find the
angle subtended by its diagonals as is shown in the diagram below. |
| Solution:
|
 |
|
|
|
|
|
|
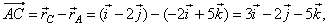| Example:
Given are points, A(-2,
-3,
1), B(3,
-1,
-4), C(0,
2, -1) and
D(-3,
0, 2), determine the scalar and
vector components of the vector AC
onto vector BD. |
| Solution:
The scalar component of the vector
AC
onto vector BD, |
|
|
|
The vector component of the vector
AC
in the direction of the vector BD
equals the product of the scalar component ACBD
and the unit vector BD°
that is
|
|
|
